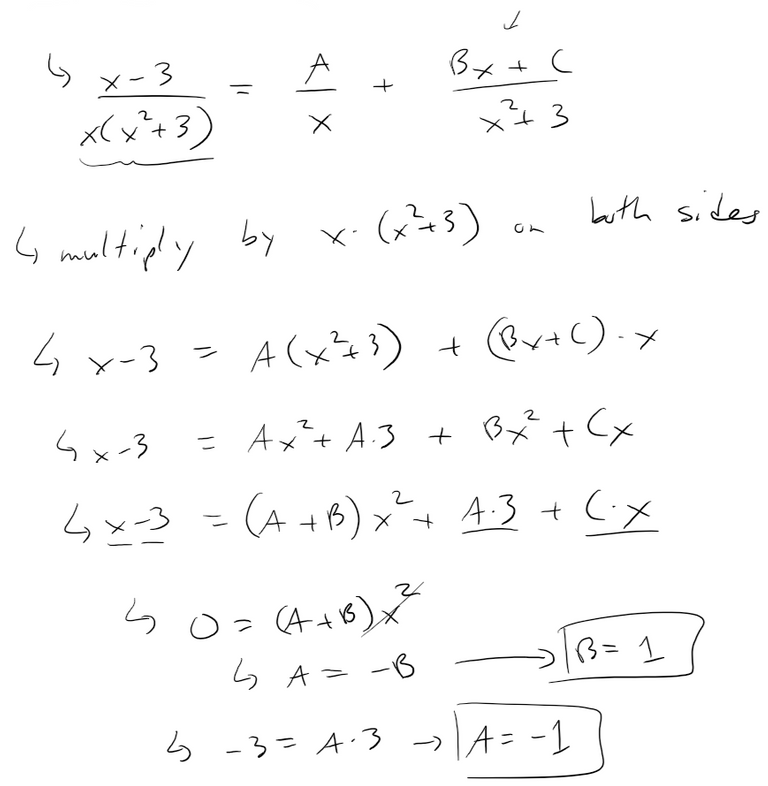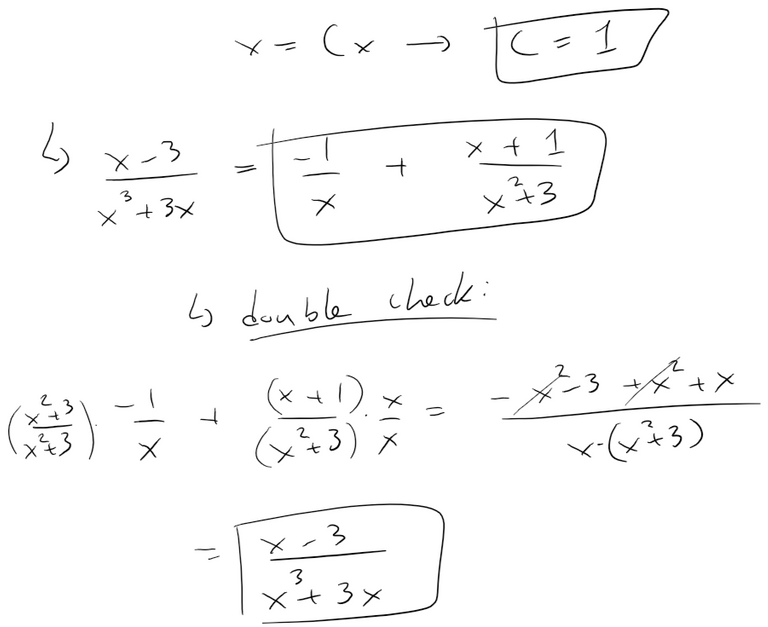In this video I go over further into partial fraction decomposition and show how to decompose rational functions that have non-linear factors (or irreducible factors) in the denominator. The method used for this case is similar to the technique used for linear factors but we have to account for the "size" of the numerator in the partial fractions so we need to apply write the numerator of each non-linear factor as a polynomial.

Watch Video On:

• DTube:
• BitChute:
• YouTube: https://youtu.be/ZK8akA4BE84

Download Video Notes: http://1drv.ms/1z2g6OG

View Video Notes Below!

Download These Notes: Link is in Video Description.
View These Notes as an Article: https://steemit.com/@mes
Subscribe via Email: http://mes.fm/subscribe
Donate! :) https://mes.fm/donate

Reuse of My Videos:

• Feel free to make use of / re-upload / monetize my videos as long as you provide a link to the original video.

Fight Back Against Censorship:

• Bookmark sites/channels/accounts and check periodically
• Remember to always archive website pages in case they get deleted/changed.

Join my private Discord Chat Room: https://mes.fm/chatroom

Check out my Reddit and Voat Math Forums:

• Reddit: https://reddit.com/r/AMAZINGMathStuff
• Voat: https://voat.co/v/AMAZINGMathStuff

Buy "Where Did The Towers Go?" by Dr. Judy Wood: https://mes.fm/judywoodbook
Follow My #FreeEnergy Video Series: https://mes.fm/freeenergy-playlist
Watch my #AntiGravity Video Series: https://steemit.com/antigravity/@mes/series

• See Part 6 for my Self Appointed PhD and #MESDuality Breakthrough Concept!

Follow My #MESExperiments Video Series: https://steemit.com/mesexperiments/@mes/list

---

NOTE #1: If you don't have time to watch this whole video:

• Skip to the end for Summary and Conclusions (If Available)
• Play this video at a faster speed.
  -- TOP SECRET LIFE HACK: Your brain gets used to faster speed. (#Try2xSpeed)
  -- Try 4X+ Speed by Browser Extensions or Modifying Source Code.
  -- Browser Extension Recommendation: https://mes.fm/videospeed-extension
  -- See my tutorial to learn more: https://steemit.com/video/@mes/play-videos-at-faster-or-slower-speeds-on-any-website
• Download and Read Notes.
• Read notes on Steemit #GetOnSteem
• Watch the video in parts.

NOTE #2: If video volume is too low at any part of the video:

• Download this Browser Extension Recommendation: https://mes.fm/volume-extension

Partial Fraction Decomposition: Non-Linear Factors

In my earlier videos I went over techniques in partial fraction decomposition for the cases where the factors were linear, such as:

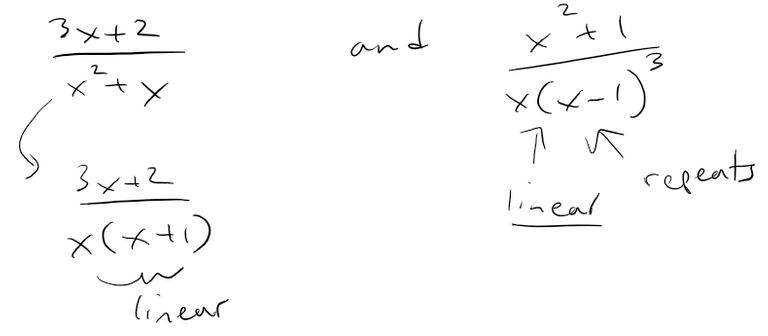
What if the factors were non-linear (irreducible)? Consider the following rational function:

Now in this case if we were to apply the same technique as previously shown, we come across a problem:

The problem is that the B coefficient is paired with a x to the power of 1 while the A coefficient is paired with a x to the power of 2. This means that one of the numerators of the partial fractions is being forced to have a higher power than the other one.

Thus we need to make both coefficients be paired with the higher power. I will detail the proof of this in a later video so stay tuned!

Basically accounting for the "size" of the numerator we modify the normal technique for partial fraction decomposition: