Integrals and Volumes: Example 4 Walled Cylinder Volume
In this video I go over another example on finding the volume of a shape using integrals. This example involves finding the volume formed by rotating the region between the functions y = x and y = x2 about the x-axis. The volume formed by rotating this region is a walled cylinder with a varying wall thickness.
Watch Video On:
- DTube: https://d.tube/#!/v/mes/QmUqqj3tSMYcieBtVLMzfFjgx9fb4CqyhDuwKVh45oTF7e
- BitChute: https://www.bitchute.com/video/sq9w6iyJOvJ6/
- YouTube: https://youtu.be/8OL6-k2Xw04
Download Video Notes: http://1drv.ms/1BbYxvr
View Video Notes Below!
Download These Notes: Link is in Video Description.
View These Notes as an Article: https://steemit.com/@mes
Subscribe via Email: http://mes.fm/subscribe
Donate! :) https://mes.fm/donateReuse of My Videos:
- Feel free to make use of / re-upload / monetize my videos as long as you provide a link to the original video.
Fight Back Against Censorship:
- Bookmark sites/channels/accounts and check periodically
- Remember to always archive website pages in case they get deleted/changed.
Join my private Discord Chat Room: https://mes.fm/chatroom
Check out my Reddit and Voat Math Forums:
Buy "Where Did The Towers Go?" by Dr. Judy Wood: https://mes.fm/judywoodbook
Follow along my epic video series:
- #MESScience: https://mes.fm/science-playlist
- #MESExperiments: https://steemit.com/mesexperiments/@mes/list
- #AntiGravity: https://steemit.com/antigravity/@mes/series
-- See Part 6 for my Self Appointed PhD and #MESDuality Breakthrough Concept!- #FreeEnergy: https://mes.fm/freeenergy-playlist
NOTE #1: If you don't have time to watch this whole video:
- Skip to the end for Summary and Conclusions (If Available)
- Play this video at a faster speed.
-- TOP SECRET LIFE HACK: Your brain gets used to faster speed. (#Try2xSpeed)
-- Try 4X+ Speed by Browser Extensions or Modifying Source Code.
-- Browser Extension Recommendation: https://mes.fm/videospeed-extension
-- See my tutorial to learn more: https://steemit.com/video/@mes/play-videos-at-faster-or-slower-speeds-on-any-website- Download and Read Notes.
- Read notes on Steemit #GetOnSteem
- Watch the video in parts.
NOTE #2: If video volume is too low at any part of the video:
- Download this Browser Extension Recommendation: https://mes.fm/volume-extension
Integrals and Volumes: Example 4 Walled Cylinder Volume
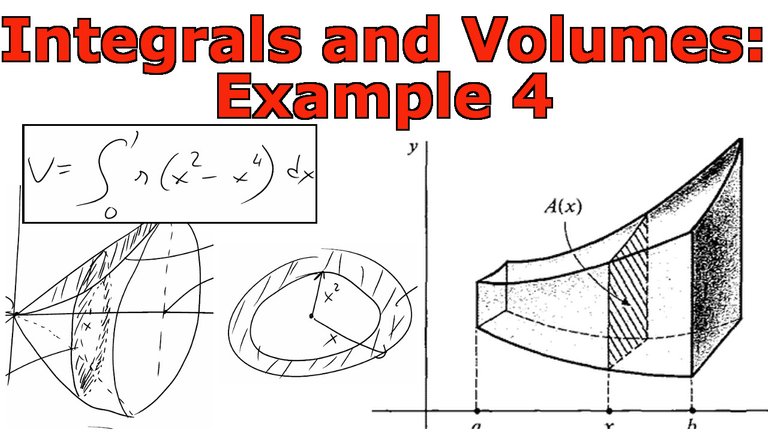
Example:
The region R enclosed by the curves y = x and x2 is rotated about the x-axis. Find the volume of the resulting solid.
Solution:

Good friends
Nice... remember my University times :)
very helpful and useful sharing. good work ;)
Math education solution easy to study
which book for analysis do You use?
I mainly follow my calculus book which can download here: