An Overview Of The Famous Number Pi (π)
Hi there. This mathematics post is dedicated to the famous irrational number pi (π). As pi starts with 3.1415926535 and then a bunch of endless digits, it is celebrated on March 14 in a calendar year. Pi is such a famous number that appears in many branches of mathematics and even outside of mathematics.
This post is an overview where I cover the pi-related topics that I know of and that I am somewhat familiar with.
Warning: This is a long post (probably a 10 to 15 minute read). It is also technical. If the technical parts do not suit you skip to the second last section on the pi memes, jokes and apparel.
Math text rendered with Quicklatex.com

Topics
- What Is Pi?
- Websites That Dedicate To Pi's Digits
- Pi In Geometry
- Pi In Trigonometry
- Calculus Appearances
- Infinite Series
- Complex Numbers & Euler's Formula
- Probability: Normal Distributions / Gaussians
- Cauchy Probability Distribution
- Monte Carlo Simulation Method To Approximate Pi: Circle Inside A Square
- Buffon's Needle Problem
- Pi Memes & Apparel
- Videos About Pi
What Is Pi?
Pi (symbol: π, pronounced like the food pie) is an irrational number that contains an endless amount of digits after the decimal place.
This value of pi represents the ratio of a circle's circumference (perimeter) to its own diameter. (Screenshot below from MathisFun website)
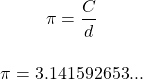

The neat part about pi (π) is that this value holds true regardless of the size of the circle with its circumference and the diameter.
Websites That Dedicate To Pi's Digits
As pi is an irrational number with infinite digits, there are people and even groups of people that try to obtain as many digits of pi as possible. Some go as far as trying to memorize as many digits as possible.
There are websites out there that do list the digits of pi. Here are a few.
- One Million Digits Of Pi
- 100,000 Digits Of Pi
- Pi to 10,000 Digits From The University of Utah
- Google just smashed the world record for calculating digits of pi
With me personally, I do not like memorizing even though I think I am okay at it. Memorizing digits of pi for me does not provide much utility for me.

Pi In Geometry
Pi is often see in geometry where we deal with circles, cylinders, cones, spheres and anything circular.
One of the first times that pi is seen is from the area and circumference of a circle.
The area of the circle is the radius squared multiplied by the value of pi.
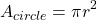
For the perimeter or circumference of the circle, we have:
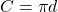
Here are the formulas for surface area (SA) and volumes (V) for cylinders, cones and spheres.
<center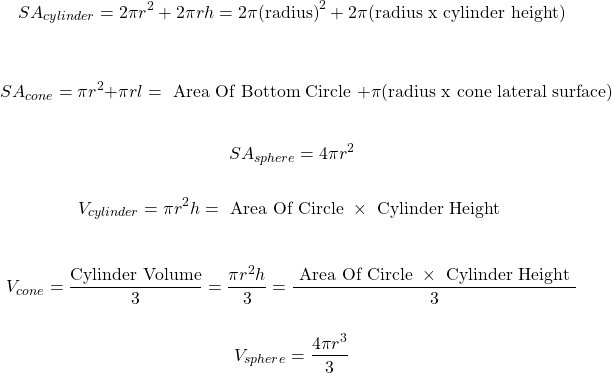
Pi In Trigonometry
Pi makes additional appearances in high school mathematics in the trigonometry section.
Degrees To Radians Measures For Angles
When it comes to measuring angles, the degrees measure is the measure of choice. At the high school level and university level, we move away from degrees and convert angles from degrees to radians.
One radian is 180 degrees divided by pi.
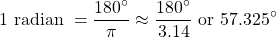
Multiplying both sides by pi gives the following important result.
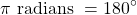
This makes pi a multi-purpose number. Pi can be represented as the number 3.14159 or from pi radians to 180 degrees.
Special Right Angle Triangles
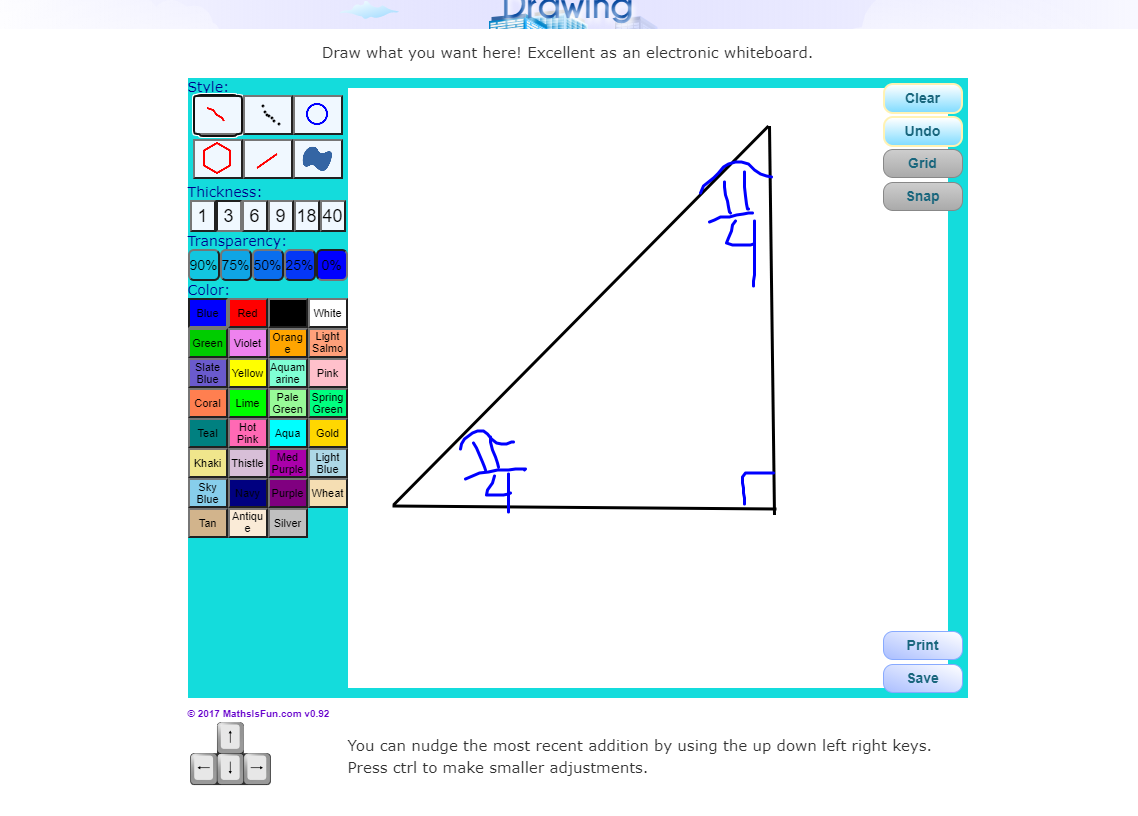
Pi does play a role when it comes to angles in special right angle triangles. I cover two right angled triangles.
With a right angled isoceles triangles, we have the lengths of the legs of 1 unit each. From the Pythagorean theorem, the hypotenuse can be determined to be sqrt(2) units. As this isoceles triangle has 180 degrees in total with a right angle, it follows that the two identical angles equal to 90 degrees. This means that each of the two angles are 45 degrees each.
In radians, the 90 degree angle is pi/2 and each 45 degree angle is pi/4.
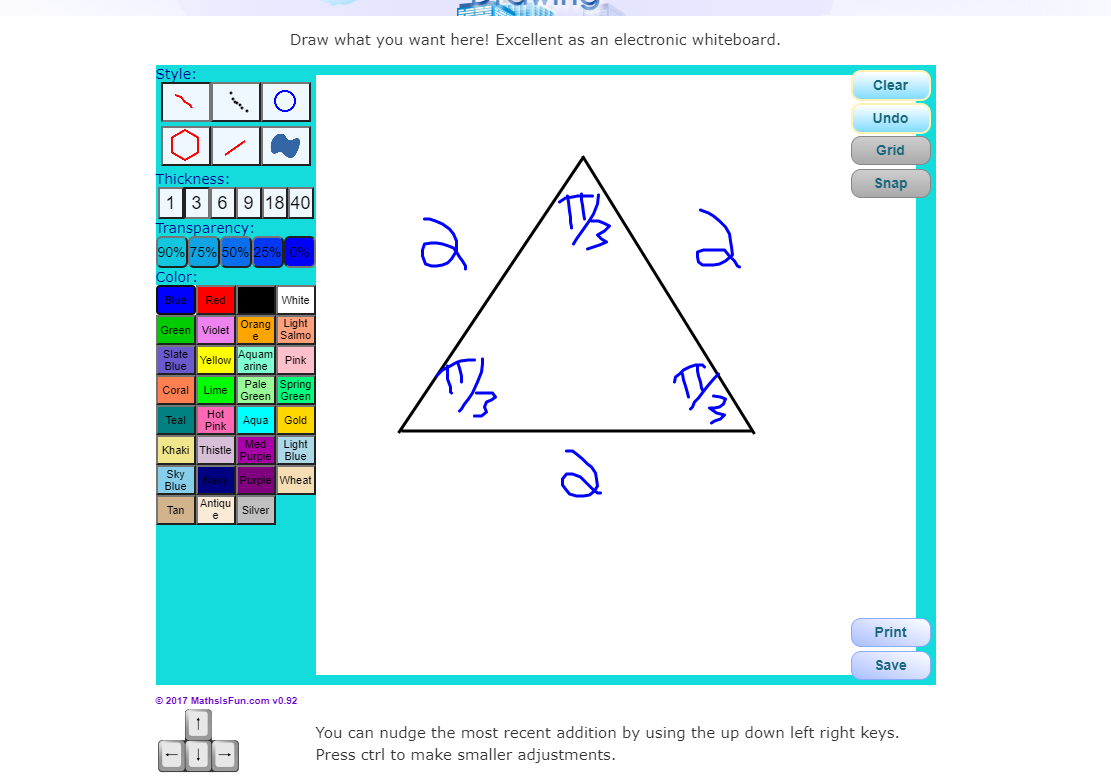
The second special right angle triangle is derived from an equilateral triangle with 2 units length on each of the three sides. Each angle in the equilateral triangle is 60 degrees or pi/3. (Screenshot below from MathisFun website)
A perpendicular bisector line is drawn from the top corner of the triangle to the base of the triangle at a 90 degree angle. This splits the equilateral triangle into two right angled triangles. It also meets the base the of the triangle at a right angle.
The smaller right angle triangle has a base length of 1 and a hypotenuse of 2 units. Usage of the Pythagorean Theorem will yield the height of the right angled triangle to be the square root of 2.
In addition, the perpendicular bisector line also splits the top angle of the triangle into two 30 degree (pi/6) angles. This makes the third angle 60 degrees or pi/3.
Here is a summary picture. (Screenshot below from MathisFun website)
Trigonometric Identities With Pi
The value of pi (π) does show up in some of trigonometric identities. Remember that π = 180° which makes 2π = 360°, π/2 = 90°.
Since 360 or 2π degrees is one whole rotation where you end up and the same spot we have these trigonometric identities. Note that k is an integer symbolizing the number of rotations counterclockwise (positive) and clockwise (negative). Theta (θ) is an angle.
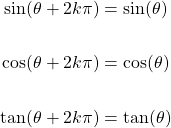
These also hold true for the cosecant, secant and cotangent reciprocal trigonometric functions.
There are more trigonometric identities that involve pi but the amount of content there would amount to another post of sorts.
Calculus Appearances For Pi
Pi does show up in calculus from integration. Here are few examples.
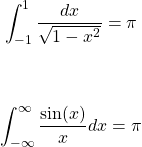
Integral Of Arctan (Tan Inverse) Over The Real Line Domain
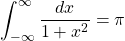
Gaussian Integral
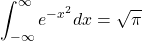
This has a more general case of:
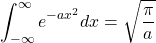
Gamma Function
The Gamma Function does have a couple of usages depending on its input. My first time seeing this Gamma function was from probability theory with the Gamma distribution.
Given a positive integer n, the gamma function is represented as a factorial.
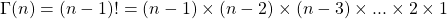
If the number put is a complex number z with a positive real number part, the gamma function is an improper integral.
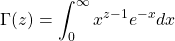
One notable result of the gamma function is 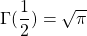 .
.
You can find more information about pi with calculus from this Wikipedia page.
Infinite Series & Pi
There are actually quite a handful of infinite series formulas which have answers involving pi. Some of them are kind of complicated. I showcase a few here.
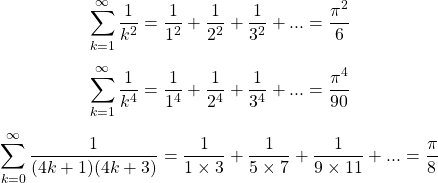
Complex Numbers & Euler's Formula
Complex numbers are an extension to the real numbers. In the real number system, taking the square root of a negative number gives an error but not in the complex numbers space.
The imaginary number i is given by  . There is also the property of
. There is also the property of  .
.
A complex number z has a real part a and an imaginary part b of the form z = a + bi.
Euler's Formula
Trigonometric functions can be related to the complex exponential function. Here is Leonard Euler's formula given a real number x:
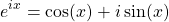
When I was taking a course on complex analysis, the notation that we used was cis(x). This is shorthand notation.
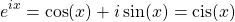
What happens if x = pi?
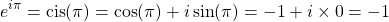
Rearranging from  , Euler's identity can be obtained.
, Euler's identity can be obtained.
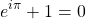
References:
- https://en.wikipedia.org/wiki/Euler%27s_formula
- https://en.wikipedia.org/wiki/Cis_(mathematics)
- https://www.mathsisfun.com/numbers/complex-numbers.html

Probability - Normal Distribution / Gaussians
The famous Normal distribution or Gaussian from probability and statistics does include the value of pi. Those who are taking a more mathematical version of probability and statistics courses will see the probability density function (p.d.f) for the Normal distribution.
A normal random variable X with a mean μ and its variance 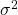 has the probability density function of:
has the probability density function of:
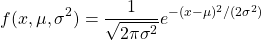
The special case of the normal distribution is the standard normal distribution. This is where the normal random variable Z has a mean μ is equal to 0 and the variance 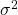 is equal to the value of 1.
is equal to the value of 1.
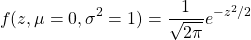
Note that the standard normal distribution is used when it comes to finding probabilities given z-scores. (A z-score is the amount of standard deviations away from the mean for a value in a numeric list of values.) When a probability is computed given a z-score, we are actually using a cumulative density function (c.d.f). This is for computing the probability or area under the curve from negative infinity to a given z-score (z).
This probability is represented as an integral for math based probability and statistics courses. Introductory statistics students will almost never see a math integral.
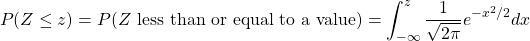
If you want the probability greater than a value 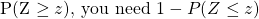 .
.
Normal Distribution Integral & Probability Equal To 1
From negative infinity to positive infinity, the area under the entire curve for the standard normal distribution is 1. Mathematically this is represented as:
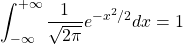
With the property of integrals, the one over the root of 2 pi can be moved outside the integral. Then we can multiply both sides by the square root of 2 pi to obtain a calculus integral result.

This last integral above follows the general form of the Gaussian function (mentioned earlier). Substitute a = 1/2 below to obtain the result above.
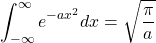
The Error Function
Another concept related to the integral above is called the error function or erf. I have seen it once and don't really use it. The function is expressed as:

References:
- https://mathworld.wolfram.com/NormalDistribution.html
- https://equplus.net/eqninfo/Equation-258.html
- https://mathworld.wolfram.com/Erf.html

Probability - Cauchy Distribution
Reference: https://www.itl.nist.gov/div898/handbook/eda/section3/eda3663.htm
The Cauchy distribution is not as well known to many versus the Normal distribution. Both the Normal distribution and the Cauchy Distribution do look similar.
Here is the generalized probability density function (p.d.f) for the Cauchy Distribution with the location parameter t and the scale parameter s.
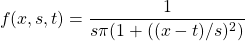
Setting t = 0 and s = 1 turns the above into the standard Cauchy distribution.
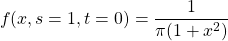
The maximum value for the standard Cauchy distribution is  which occurs at
which occurs at x = 0.
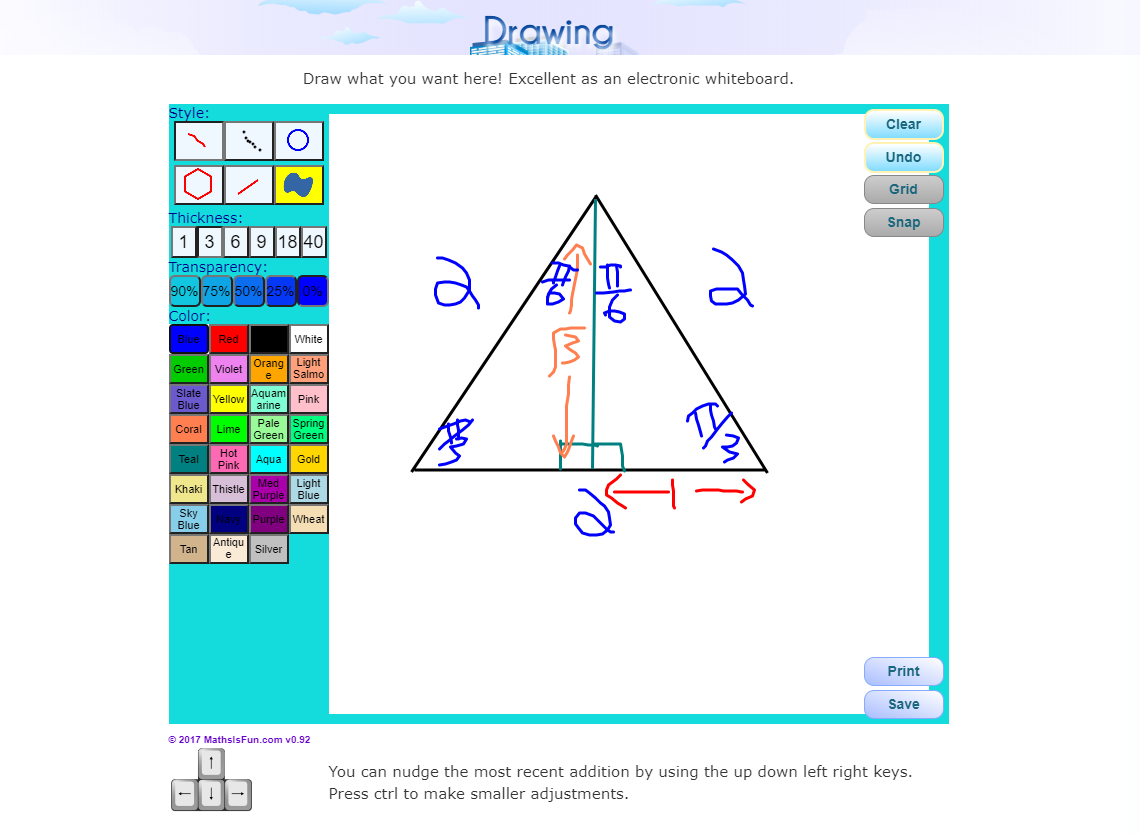
Monte Carlo Simulation Method To Approximate Pi: Circle Inside A Square
An approximate value of pi can be obtained with the use of Monte Carlo Simulations from the probability and statistics field. This particular Monte Carlo method uses simulated or sampled random variables to help with the estimation for the value of pi.
This simulation involves a square with 2 units in length. The points of the square are at (-1, 1), (1, 1), (-1, -1) and (1, -1). Add a circle of radius 1 centered at the origin inside the square. (Desmos screenshot)
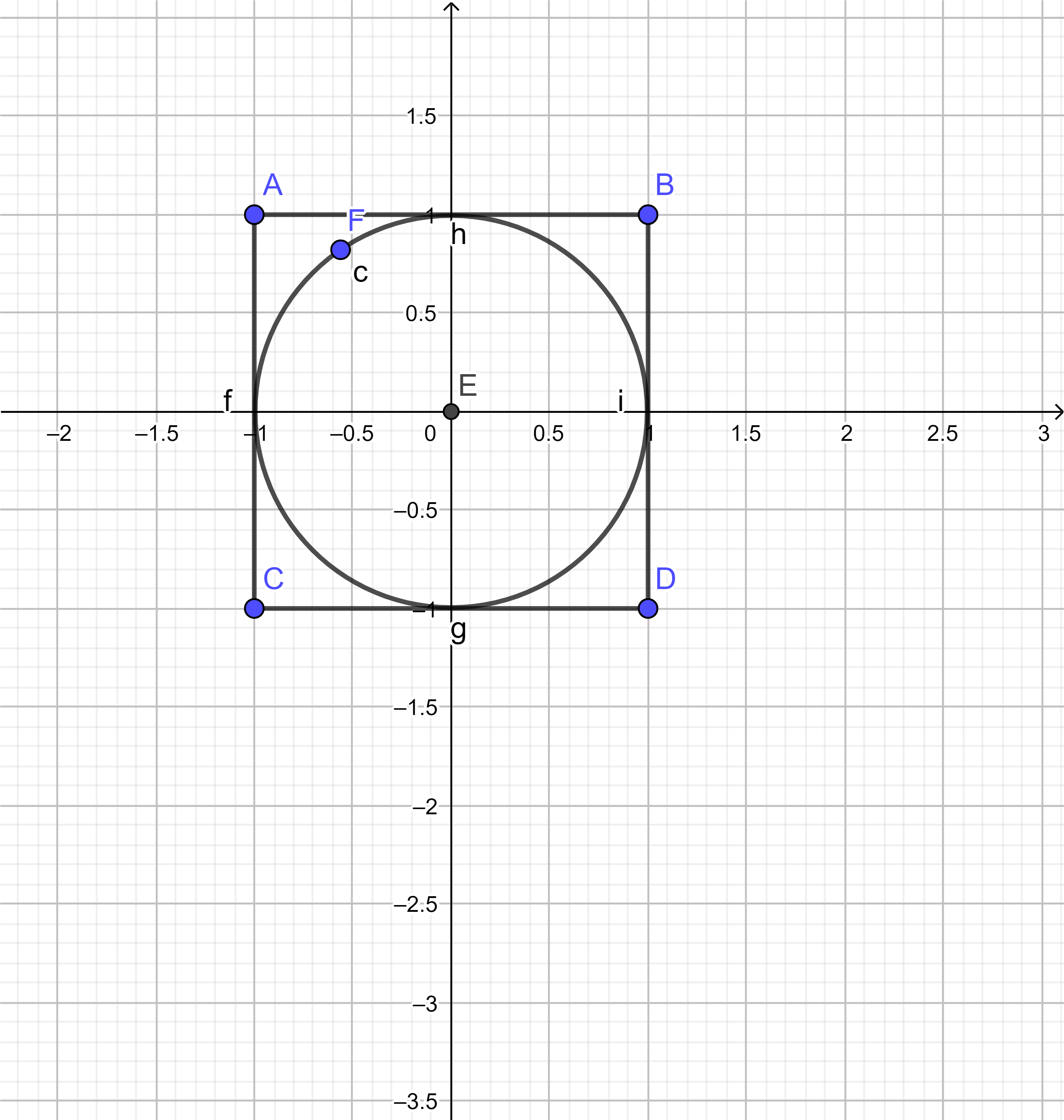
The area of the unit circle is simply pi while the area of the square is 4 square units. Dividing the area of the unit circle by the area of the square gives pi/4. This pi/4 value is what needs to be estimated.
Monte Carlo Simulation For Approximating Pi
In a given trial here are the steps.
Step One
Simulate 2 Uniform Random Variables from -1 to 1. The first uniform random variable is for the x-coordinate and the second uniform random variable is for the y-coordinate. That is 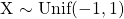 and
and 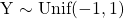 .
.
Step Two
If  then the generated (X, Y) point is in the unit circle. If not, then that point is outside the circle but in the square.
then the generated (X, Y) point is in the unit circle. If not, then that point is outside the circle but in the square.
Step Three
Run the above two steps, until all the n trials are done.
After n trials, the ratio of interest is:
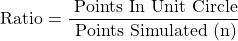
This ratio is an estimate for  . As more trials are done, the ratio gets closer to the value of
. As more trials are done, the ratio gets closer to the value of  . You could do 4 times the ratio to estimate pi itself.
. You could do 4 times the ratio to estimate pi itself.
I have a simple app done with R programming and RShiny that does this simulation.
Buffon's Needle Problem
Reference: https://mathworld.wolfram.com/BuffonsNeedleProblem.html
This problem is quite technical. When I first heard about it, I got confused. I am not the best of explaining the entire details of it so I add a supplementary Youtube video for this.
You have equally spaced parallel lines that are spaced evenly with a distance of let's say d units. The objective is to find the probability that a needle of length l will land on one of the parallel lines. A needle on the floor may not be positioned in the same way.
Here is one Youtube video (shown below) that explains the problem and the solution. The video use a needle of length one and two units between the parallel lines. The answer does involve pi. In fact, the probability of the needle crossing the line is  .
.
Pi Memes, Jokes & Apparel
There are people who love pi (π) love so much, they have made jokes, memes and apparel associated with pi. Although I do not own a shirt from them, Snorg Tees have some neat shirts with pi on it.
Here are a few links that contain pi jokes.
- https://www.rd.com/list/corny-math-jokes-pi-day/
- https://www.distractify.com/p/pi-day-memes
- https://nineplanets.org/pi-jokes/
Videos About Pi
As I am one guy, I cannot cover everything about pi. Here are some videos on pi.
- Math Antics - Circles, What Is PI?
- The Pi Song (Memorize 100 Digits Of π) | SCIENCE SONGS
- 3 Ways Pi Can Explain Practically Everything
- The Most Unusual Ways Pi Shows Up In Mathematics | Can You Explain These?
- A Brief History of Pi
That is my overview of the number π. This number shows up in so many places in mathematics. More than you think.


somebody called my name? Oh no, it's about my positive brother :D
great post, you've compiled a lot of details in here!
Thank you. I am glad you enjoyed this one. Enjoy your birthday (π Day).
Just in time for pi day!
This was the plan. :)
Thanks for your contribution to the STEMsocial community. Feel free to join us on discord to get to know the rest of us!
Please consider supporting our funding proposal, approving our witness (@stem.witness) or delegating to the @stemsocial account (for some ROI).
Please consider using the STEMsocial app app and including @stemsocial as a beneficiary to get a stronger support.