Precise Definition of a Limit - Example 3
In this video I continue with the wonderful world of limits and go over a more difficult example using the precise definition of a limit. In this case I show how to prove the limit of x2 as x approaches 3 is equal to 9 using a pretty clever method. For more complicated functions, using the precise definition to prove limits becomes increasingly more difficult. But luckily we can simply prove them using the limit laws which I went over in my earlier videos (see video links below). But those limit laws need to be proven and I will prove each one in my videos to come.
Watch video on:
- BitChute: https://www.bitchute.com/video/9kw6KM8pyhcC/
- 3Speak:
- DTube: https://d.tube/#!/v/mes/34j39de99dc
- YouTube: https://youtu.be/7xDC8aV-mMg
Download video notes: https://1drv.ms/b/s!As32ynv0LoaIiMxqJcCXle9g2lOtIQ?e=dYvf7M
View Video Notes Below!
Download these notes: Link is in video description.
View these notes as an article: https://peakd.com/@mes
Subscribe via email: http://mes.fm/subscribe
Donate! :) https://mes.fm/donate
Buy MES merchandise! https://mes.fm/storeReuse of my videos:
- Feel free to make use of / re-upload / monetize my videos as long as you provide a link to the original video.
Fight back against censorship:
- Bookmark sites/channels/accounts and check periodically
- Remember to always archive website pages in case they get deleted/changed.
Buy "Where Did The Towers Go?" by Dr. Judy Wood: https://mes.fm/judywoodbook
Subscribe to MES Truth: https://mes.fm/truthJoin my forums!
- Hive community: https://peakd.com/c/hive-128780
- Reddit: https://reddit.com/r/AMAZINGMathStuff
- Discord: https://mes.fm/chatroom
Follow along my epic video series:
- #MESScience: https://mes.fm/science-playlist
- #MESExperiments: https://peakd.com/mesexperiments/@mes/list
- #AntiGravity: https://peakd.com/antigravity/@mes/series
-- See Part 6 for my Self Appointed PhD and #MESDuality breakthrough concept!- #FreeEnergy: https://mes.fm/freeenergy-playlist
- #PG (YouTube-deleted series): https://peakd.com/pg/@mes/videos
NOTE #1: If you don't have time to watch this whole video:
- Skip to the end for Summary and Conclusions (if available)
- Play this video at a faster speed.
-- TOP SECRET LIFE HACK: Your brain gets used to faster speed!
-- Browser extension recommendation: https://mes.fm/videospeed-extension
-- See my tutorial to learn more: https://peakd.com/video/@mes/play-videos-at-faster-or-slower-speeds-on-any-website- Download and read video notes.
- Read notes on the Hive blockchain #Hive
- Watch the video in parts.
-- Timestamps of all parts are in the description.Browser extension recommendations:
- Increase video audio: https://mes.fm/volume-extension
- Text to speech: https://mes.fm/speech-extension
Precise Definition of a Limit – Example 3
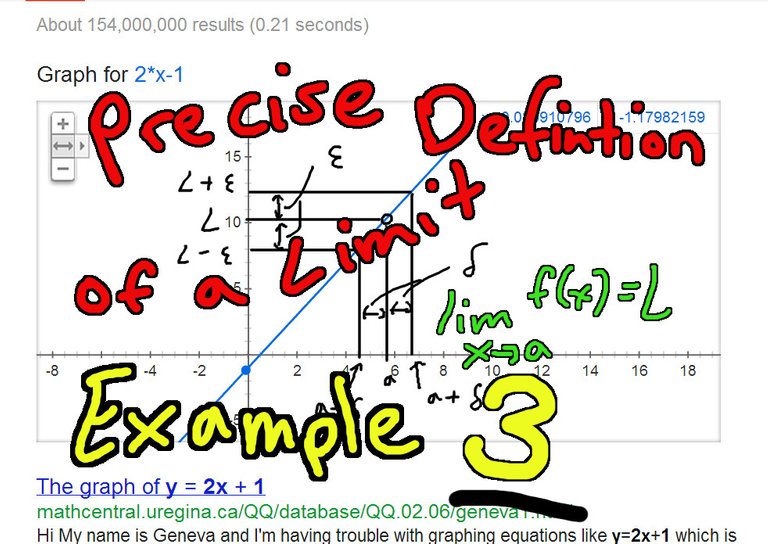
Precise Definition
Let f be a function defined on some open interval that contains the number ‘a’, except possibly at ‘a’ itself.
Then we can say that the limit of f(x) as x approaches ‘a’ is ‘L’, and we write:
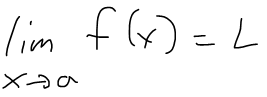
If for every number ε > 0 there is a number δ > 0 such that:

Example
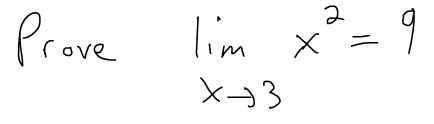
Solution
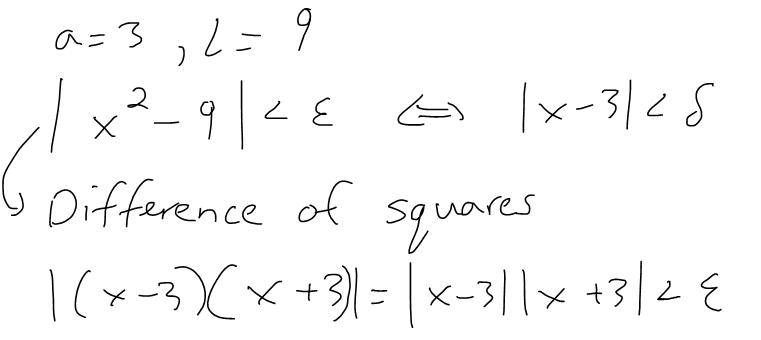
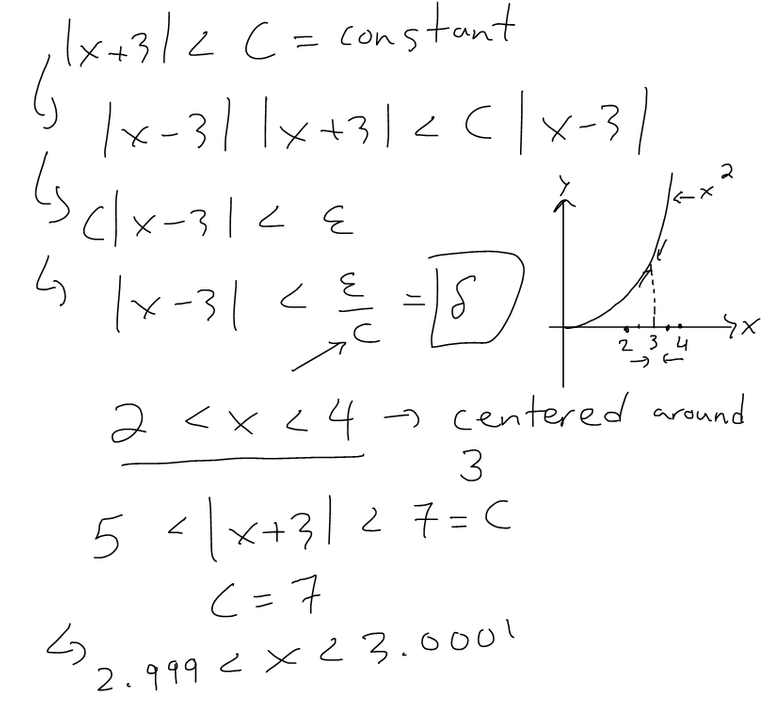

Important Notes From This Example
- Not always easy to prove the limit using the precise definition of a limit
- In fact, complicated functions like f(x) = (6x2 – 8x + 9)/(2x2 – 1) require a great deal of ingenuity
- Fortunately, we can actually prove limits such as these using the Limit Laws which I covered earlier
- All we need to do first is prove each limit law using the precise definition of a limit (in my later videos)
https://twitter.com/MathEasySolns/status/1358987573419384833