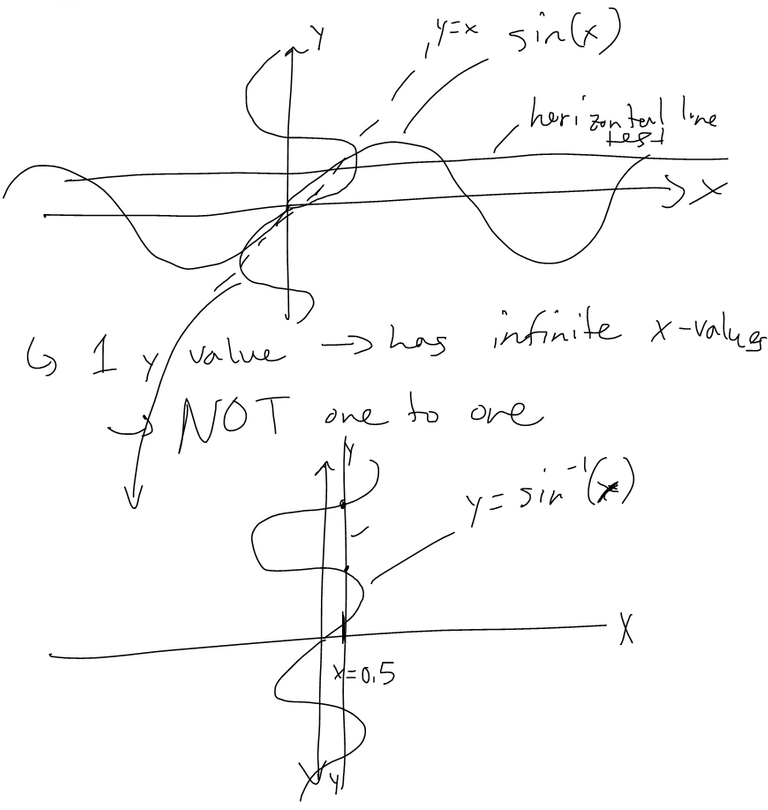
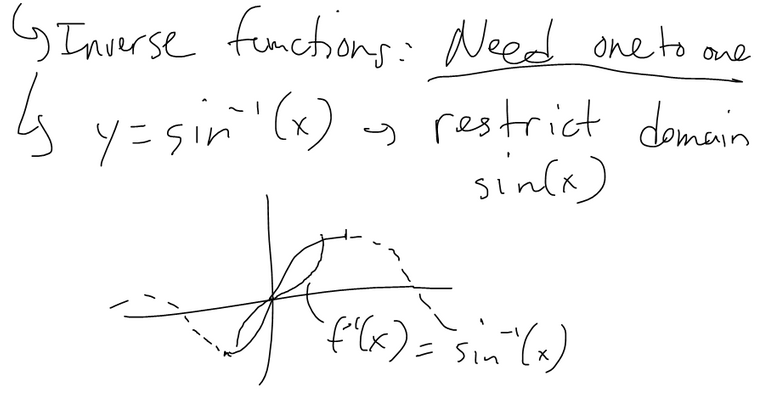
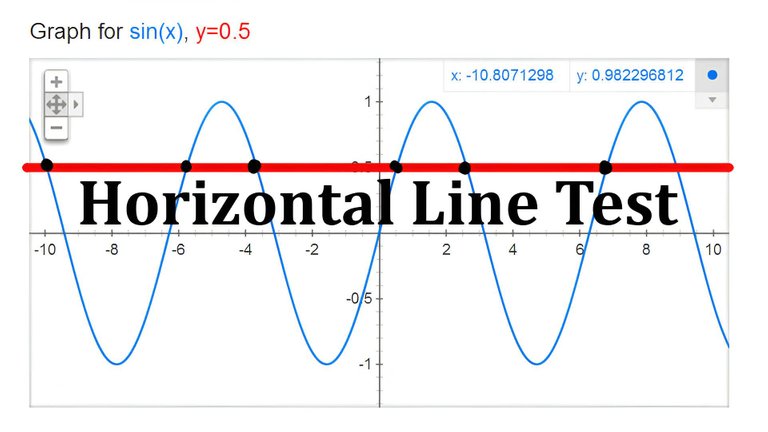
In this video I clarify the inverse functions in which I defined in my earlier video to state that it is only applicable to one to one functions. This requirement is illustrated in trigonometric functions which are not one to one and there inverse would require that an x value have multiple y values. I allude to how we avoid this restriction by restricting the domains of trig functions.

Download video notes: https://1drv.ms/b/s!As32ynv0LoaIiekrGIx4KdpjXIprGg?e=KnDG6f

Watch video on:

• 3Speak:
• Odysee: https://odysee.com/@mes:8/Inverse-Functions-Part-2-One-to-One-Functions-and-Horizontal-Line-Test:e
• BitChute: https://www.bitchute.com/video/SM6vbY1E6pm1/
• Rumble:
• DTube: https://d.tube/#!/v/mes/n4svapvokq9
• YouTube: https://youtu.be/iSNuplUWrbA

View Video Notes Below!

Download these notes: Link is in video description.
View these notes as an article: https://peakd.com/@mes
Subscribe via email: http://mes.fm/subscribe
Donate! :) https://mes.fm/donate
Buy MES merchandise! https://mes.fm/store
More links: https://linktr.ee/matheasy
Follow my research in real-time on my MES Links Telegram: https://t.me/meslinks
Subscribe to MES Truth: https://mes.fm/truth

Reuse of my videos:

• Feel free to make use of / re-upload / monetize my videos as long as you provide a link to the original video.

Fight back against censorship:

• Bookmark sites/channels/accounts and check periodically
• Remember to always archive website pages in case they get deleted/changed.

Recommended Books:

• "Where Did the Towers Go?" by Dr. Judy Wood: https://mes.fm/judywoodbook

Join my forums!

• Hive community: https://peakd.com/c/hive-128780
• Reddit: https://reddit.com/r/AMAZINGMathStuff
• Discord: https://mes.fm/chatroom

Follow along my epic video series:

• #MESScience: https://mes.fm/science-playlist
• #MESExperiments: https://peakd.com/mesexperiments/@mes/list
• #AntiGravity: https://peakd.com/antigravity/@mes/series
  -- See Part 6 for my Self Appointed PhD and #MESDuality breakthrough concept!
• #FreeEnergy: https://mes.fm/freeenergy-playlist
• #PG (YouTube-deleted series): https://peakd.com/pg/@mes/videos

---

NOTE #1: If you don't have time to watch this whole video:

• Skip to the end for Summary and Conclusions (if available)
• Play this video at a faster speed.
  -- TOP SECRET LIFE HACK: Your brain gets used to faster speed!
  -- MES tutorial: https://peakd.com/video/@mes/play-videos-at-faster-or-slower-speeds-on-any-website
• Download and read video notes.
• Read notes on the Hive blockchain $HIVE
• Watch the video in parts.
  -- Timestamps of all parts are in the description.

Browser extension recommendations:

• Increase video speed: https://mes.fm/videospeed-extension
• Increase video audio: https://mes.fm/volume-extension
• Text to speech: https://mes.fm/speech-extension
  --Android app: https://mes.fm/speech-android

Inverse Functions Part 2: One to One Functions and the Horizontal Line Test

Inverse Functions

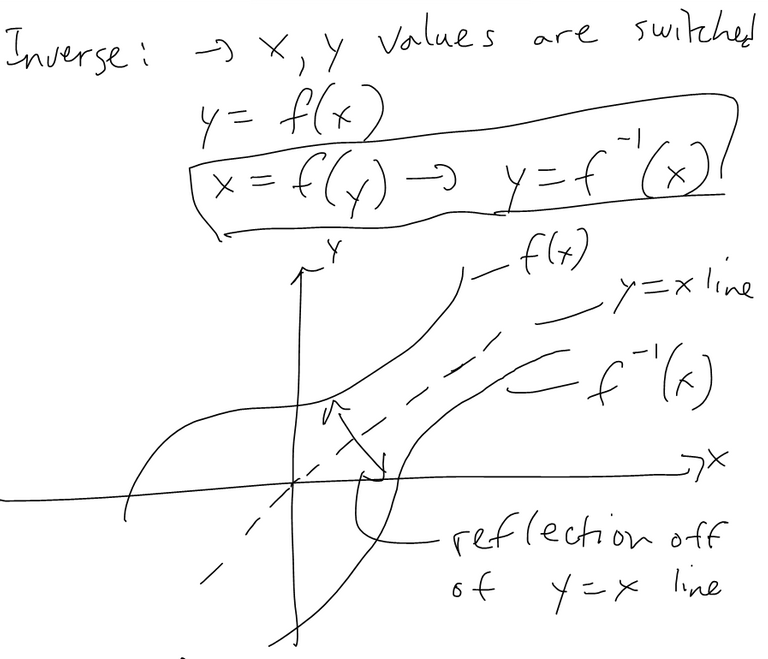
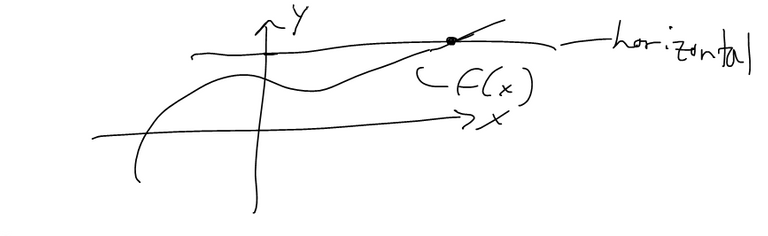
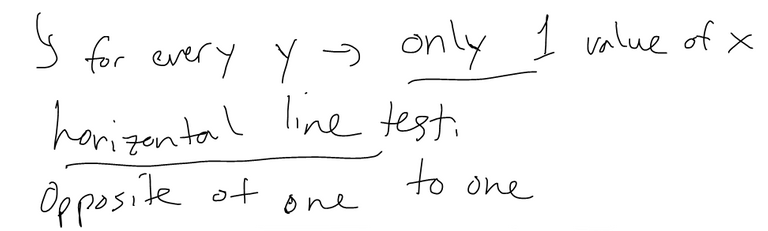
One to One Functions