26-10-2024 - Analytic geometry - dimension of a vector space [EN]-[IT]

~~~ La versione in italiano inizia subito dopo la versione in inglese ~~~

ENGLISH
26-10-2024 - Analytic geometry - dimension of a vector space [EN]-[IT]

With this post I would like to give a short instruction about the topic mentioned in the subject
(code notes: X_090)
Dimension of a vector space
The dimension of a vector space is usually indicated as dim(V)

To better understand things, let's start from the concept regarding the dimension of a vector space. The dimension of a vector space is a measure of how big a vector space is. The dimension of a vector space will also give useful information to understand how many bases there are for vector spaces
Here below I show how the dimension of a vector space is indicated.

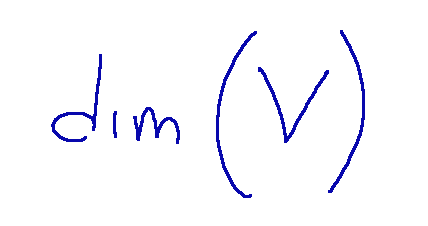
The above acronym indicates the dimension of a finitely generated vector space V which is the number of elements of any of its bases.

Let's now consider the following acronym

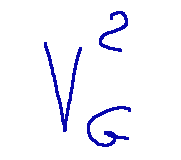
From this description we understand that the dimension is 2 and that [i,j] is a basis with 2 elements

Let's now consider the following acronym

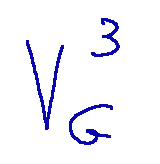
From this description we understand that the dimension is 3 and that [i,j,k] is a basis with 3 elements

Examples
As an example, below I try to ask two questions.
Is it possible to find three linearly independent geometric vectors in the following dimension?

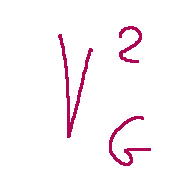
Answer
NO

It is possible to find two geometric vectors that generate the following size?

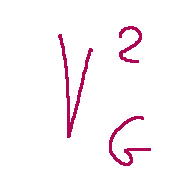
Answer
YES

Vector basis
The dimension of the vector basis is the number of linearly independent vectors {v1,...,vn} of the basis

Where:
n = the cardinality.

The basis has a dimension equal to a finite number n, that is, it is composed of n vectors.
The basis has a dimension equal to infinity, that is, it is composed of infinite vectors.

Conclusions
The dimension of a vector space is a fundamental concept in linear algebra and analytic geometry, and represents the number of vectors in a basis of that space. In practical terms, the dimension of a vector space tells us how many independent vectors are needed to describe all the vectors in the space

Question
Have you ever studied the concept of dimension of a vector space in school?

[ITALIAN]
26-10-2024 - Geometria analitica - dimensione di uno spazio vettoriale [EN]-[IT]
Con questo post vorrei dare una breve istruzione a riguardo dell’argomento citato in oggetto
(code notes: X_090)
Dimensione di uno spazio vettoriale
La dimensione di uno spazio vettoriale di solito è indicata come dim(V)

Per comprendere meglio le cose partiamo dal concetto che riguarda la dimensione di uno spazio vettoriale. La dimensione di uno spazio vettoriale è una misura di quanto è grande uno spazio vettoriale. La dimensione di uno spazio vettoriale darà informazioni utili anche per capire quante sono le basi degli spazi vettoriali
Qui di seguito mostro come è indicata la dimensione di uno spazio vettoriale.

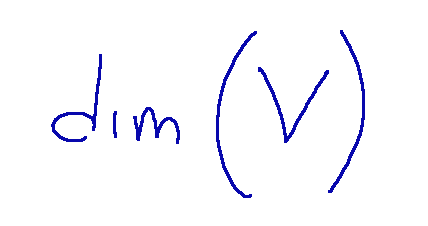
La sigla qui sopra indica la dimensione di uno spazio vettoriale finitamente generato V che è il numero degli elementi di una qualsiasi delle sue basi.

Prendiamo ora in considerazione la seguente sigla

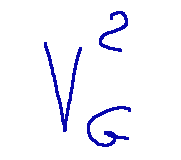
Da questa descrizione comprendiamo che la dimensione è 2 e che [i,j] è una base con 2 elementi

Prendiamo ora in considerazione la seguente sigla

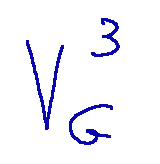
Da questa descrizione comprendiamo che la dimensione è 3 e che [i,j,k] è una base con 3 elementi

Esempi
Come esempio, qui di seguito provo a fare due domande.
È possibile trovare tre vettori geometrici linearmente indipendenti nella seguente dimensione?

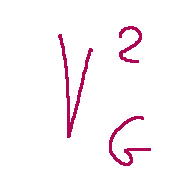
Risposta
NO

È possibile trovare due vettori geometrici che generano la seguente dimensione?

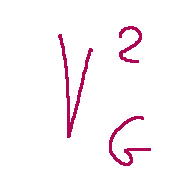
Risposta
SI

Base vettoriale
La dimensione della base vettoriale è il numero dei vettori {v1,...,vn} linearmente indipendenti della base

Dove:
n = la cardinalità.

La base ha una dimensione pari a un numero finito n, ossia è composta da n vettori.
La base ha una dimensione pari a infinito, ossia è composta da infiniti vettori.

Conclusioni
La dimensione di uno spazio vettoriale è un concetto fondamentale in algebra lineare e geometria analitica, e rappresenta il numero di vettori in una base di quello spazio. In termini pratici, la dimensione di uno spazio vettoriale ci dice quanti vettori indipendenti servono per descrivere tutti i vettori dello spazio

Domanda
Avete mai studiato a scuola il concetto di dimensione di uno spazio vettoriale?

THE END