The Role of Gamma Function in Quantum Mechanics | ChemFam #79 |

Greetings to everyone! In the last post, we have studied normalization of wavefunction and discussed postulates of quantum mechanics. Today, we shall study a mathematical gem that plays a crucial role in quantum mechanics, that is the gamma function. Initially introduced by Leonhard Euler in the 18th century, the gamma function has found its way into various branches of mathematics and physics, with quantum mechanics being no exception.

The Gamma Function:

The gamma function, denoted by Γ(x), is an extension of the factorial function to complex numbers. It is defined for all complex numbers except for non-positive integers, where it diverges. The gamma function is expressed as:

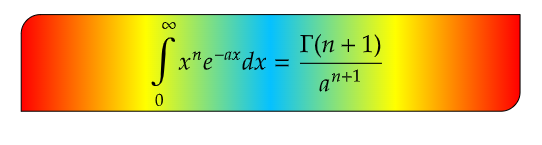
Where a is any constant. Keep in mind that this expression is valid for limits from 0 to ∞ as can be seen from the above expression.

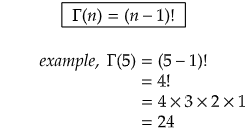
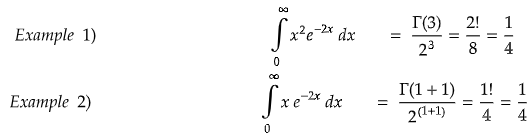
Now what if the boundary limit is from -∞ to +∞ ?

In that condition, we find two values of the gamma function depending on whether the value of n is odd or even. If n is an odd entity (Number), then the gamma function value becomes zero. If it is even, we get a non zero value. For the said limits, gamma function is expressed as:

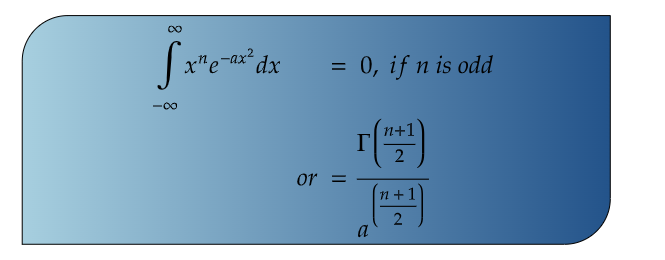
Some important gamma function values:

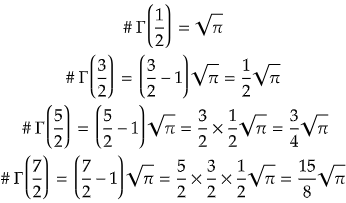
This seemingly abstract mathematical construct proves to be remarkably useful in quantum mechanics, where it aids in solving complex problems involving wavefunctions, probability amplitudes, and the energy levels of quantum systems.

Quantum Mechanics and the Schrödinger Equation:

The Schrödinger equation is the cornerstone of quantum mechanics, describing the evolution of a quantum system over time. The time-independent Schrödinger equation for a non-relativistic particle can be written as:

Ĥψ=Eψ

Here,

Ĥ represents the Hamiltonian operator,

ψ is the wavefunction of the particle, and

E is the energy of the system. The Hamiltonian operator is often expressed in terms of spatial derivatives and potential energy terms. We shall discuss the Schrodinger equation in a later post, as of now, to move to the next point in gamma function, we only needed the basics. So, do not worry.

Gamma Function in Solving the Schrödinger Equation:

In certain quantum systems, the solutions to the Schrödinger equation involve the gamma function. For example, when dealing with radial wavefunctions in spherically symmetric potentials, the radial part of the Schrödinger equation may lead to equations with the gamma function.

We can show the use of gamma function while solving the radial part by taking an example.

Problem) Write the normalized wavefunction for 1D SHO (Simple Harmonic Oscillator) if,
Ψ = e^(-βx2/2) with limits from -∞ to +∞.

Solution

I hope you have not forgotten to find out the normalization constant. (Read my previous post again if so).

So, we have the normalization constant as,

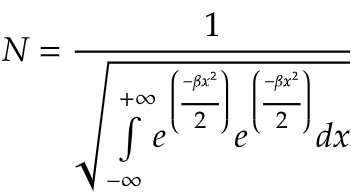
Now, we need to solve the integral and find out the normalization constant.

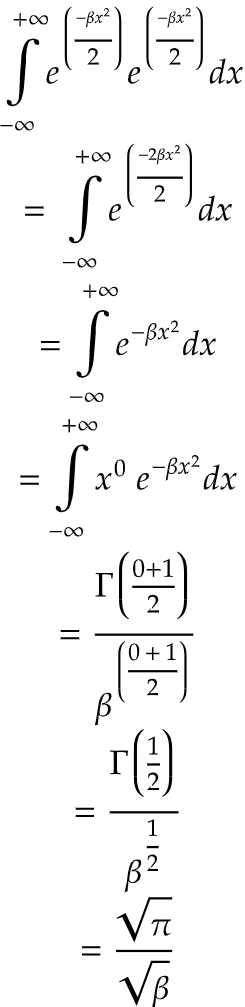
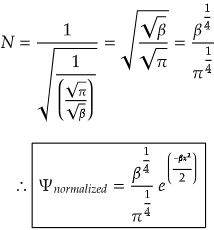
Problem) Calculate the value of N if Ψ = e^-r'a₀ (0<r<∞) for H atom.

Solution

We can solve this problem by the same method as discussed above.

We have to consider only radial part as only r limit is given.
We know, volume element for radial part is,

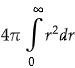
Now, the normalization constant is,

On solving the integration,

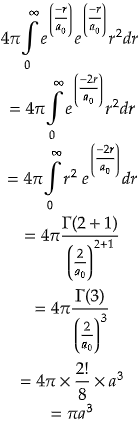
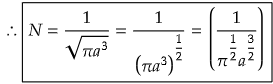
What we learnt?

• We studied what gamma function are and their mathematical interpretation. The gamma function, initially introduced for purely mathematical purposes, finds itself playing a pivotal role in describing the quantum world.

• Discussed briefly the Schrodinger equation.

• Studied the role of gamma function in solving radial part of the Schrodinger equation. The gamma function also makes an appearance when solving the Schrödinger equation for the quantum harmonic oscillator.

Software used:

The mathematical equations are prepared using mathcha.io editor.

---

Read My Previous Blogs:

---

Postulates of Quantum Mechanics and Normalization of Wavefunction |ChemFam #78|

Understanding Commutator Relations and Exploring Eigenfunctions in Quantum Mechanics |ChemFam #77|

How to find Expression of an Operator and Commutation Relations |ChemFam #76|

Basics to Quantum Chemistry: Operators, Functions and Properties of Operators |ChemFam #75|

Exploring Total Differentials and Cyclic Rules as Mathematical Maestros in Thermodynamics |ChemFam #74|

A Comprehensive Study of Euler's Reciprocal Rule in Thermodynamics |ChemFam #73|

A Deep Dive into Nutrition Essentials: Your Path to a Healthier, Happier You |ChemFam #72|

Decoding Liver Function Tests through Chemistry |ChemFam #71|

Understanding the Dynamic Roles of Metalloenzymes and Metal-Activated Enzymes |ChemFam #70|

Cracking the Thermal Code: Differential Thermal Analysis in Modern Research |ChemFam #69|

Applications and Importance of IR Spectroscopy: Shedding Light on Molecular Structures |ChemFam #68|

The Silent Revolution: How Polymers are Shaping Our World? |ChemFam #67|

Beyond the Bin: The Many Faces of Plastic Management |ChemFam #66|

Spectrophotometry Simplified: The Beer-Lambert Law in Spectrophotometry |ChemFam #65|

Chromatography: Unraveling the Science of Separation |ChemFam #64|

Colorful Clues: The Magical World of Chemical Indicators |ChemFam #63|

Colloids in Action: Impacting Your Daily Life More Than You Think |ChemFam #62|

The Complex Landscape of Opioid Analgesics: Addressing The Concerns |ChemFam #61|

Genetic Engineering: Pioneering Progress or Ethical Predicament? |ChemFam #60|

The Guardians Against Microbial Menace: Antibacterial Agents |ChemFam #59|

The Cholesterol Conundrum: The Story of Statins |ChemFam #58|

Unveiling The Control Of Chemistry: How Hormones Dictate Our Mood |ChemFam #57|

Thermodynamic Versus Kinetic Control of Reactions |ChemFam #56|

Bosons: The Quantum Glue That Holds The Universe Together |ChemFam #55|

PS The thumbnail image is being created by me using canva.com

---

Thanks for stopping by :)