Greetings again my dear friends I hope you are all very well, at any moment of our existence we can rely on the learning provided by the field of science in every way, we can be in a certain space-time and be able to observe around us a lot of information provided by our natural environment that surrounds us, information collected and standardized by man through its powerful tool of transformation and generation of knowledge as it is science.

Generally, we are on a flat surface at sea level or even below sea level, but at other times we may find ourselves at a height well above sea level, for example, on top of a mountainous formation and where atmospheric conditions vary in relation to pressure and temperature, these variables can help us to determine the height of the mountainous structure.

Exercise

A professional hiker decides to climb to the top of a mountain that borders the sea, it is his passion to be able to feel the air currents at such a high altitude and, in addition, it is his job to determine the altitude of each of the mountain structures he decides to conquer, When he is at the top of the mountain he tells his teammate that he is at the lowest part of the mountain, that the pressure at that altitude is 534 mm of Hg (millimeters of mercury) and that the air density is 1.75 g/liter, noting that the pressure at sea level is 760 mm of Hg, then, answer the following question:

a.- What would be the height of the mountain above sea level?

Solución

Data:

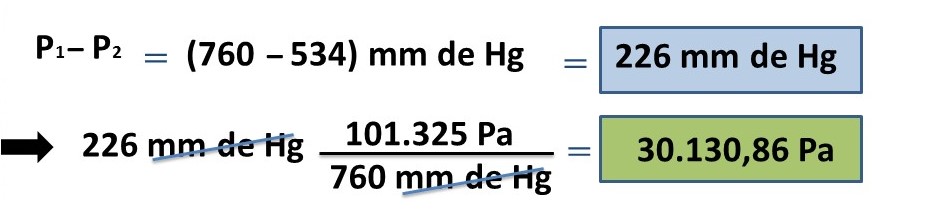
P1 = 760 mm de Hg (Sea level pressure).
P2 = 534 mm de Hg (Pressure at the top of the mountain).
d = 1,75 g/ liter (Air density).
g = 9,8 m/s2 (Gravity acceleration).
h = ? (Mountain height).

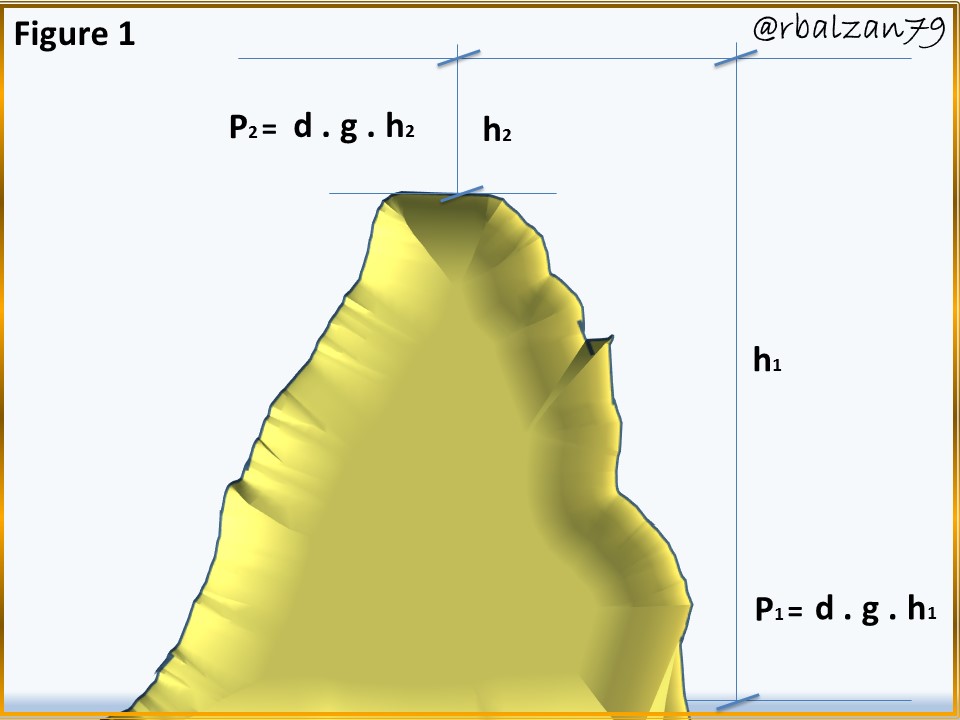
a.- Before starting to solve our question, it is very important to emphasize that all fluids (liquid and gaseous), will exert a certain pressure on the walls of any container containing them and also on those bodies that are within the action of these fluids, this force or pressure will always act perpendicular to these surfaces analyzed, therefore, the pressure (P) exerted by a certain fluid of density (d) on any point located at a certain depth (h) of the surface, will be numerically similar to that pressure exerted by a certain column of fluid of height (h), these variables are not provided by the fundamental theorem of hydrostatics by means of the following algebraic formulation:

Thus, as we wish to calculate the height of the mountain formation in Figure 1, then:

Therefore, we apply the fundamental theorem of hydrostatics at sea level and, also at the height of the mountain where the hiker arrives, then, looking at figure 1, we can determine:

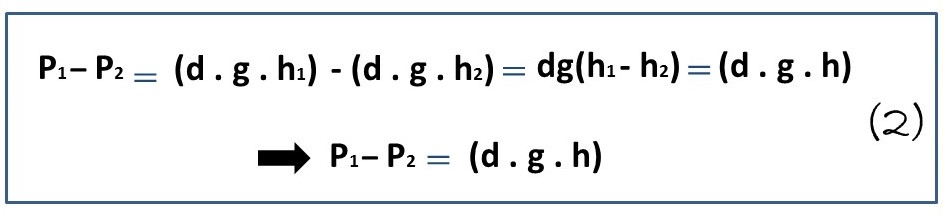
Then from formula 2, we clear our requested unknown, i.e., height (h), therefore, we have:

Therefore:

Now we transform the density of the air in this space into units of the international system:

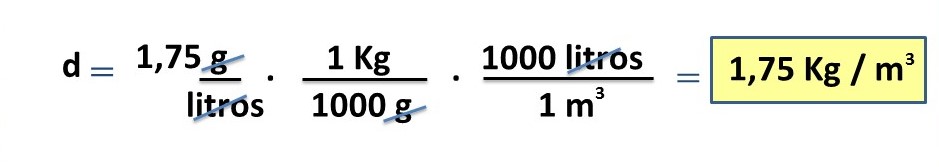
In this way we proceed to substitute the values in the formula 2 and, in this way, find the requested height.

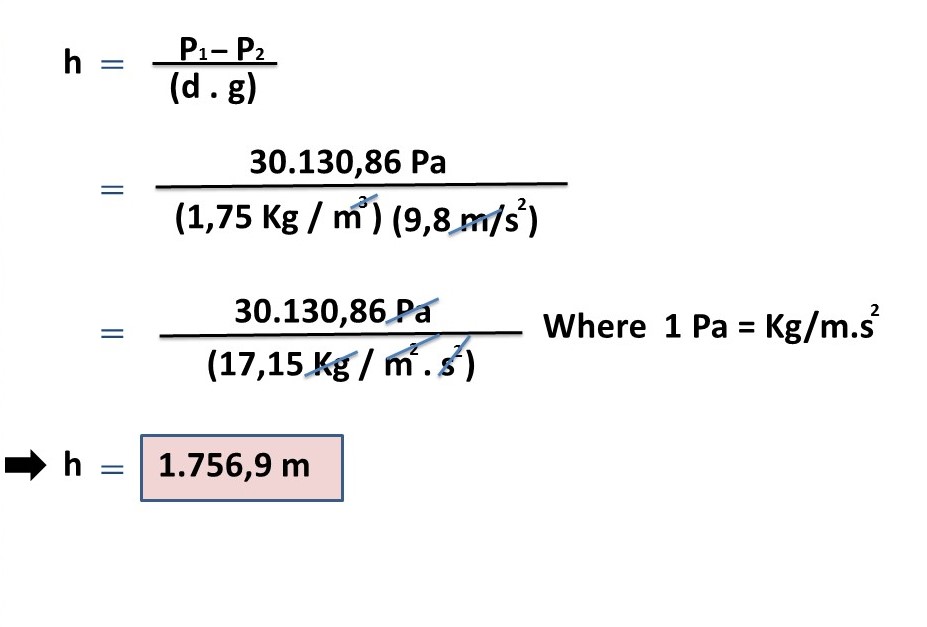
We were able to find the height of the mountain where the professional hiker climbed to the top, therefore, he managed to climb to a height of 1,756.9 m.

Conclusion

Many of us undoubtedly hear at every moment the reference of the sea level and, this in order to search or find or establish some kind of height of a certain location or mountain structures, among others, the truth is that with some data, it is possible to know the altitude of the above mentioned examples, as we noticed in the development of our exercise, where, through the implementation of some mathematical formulations we managed to know the height of a particular mountain.

It is always important to know that we can apply these mathematical formulations and make them very practical at the time of explaining any event that is around us, in this way we continue to verify that everything around us is expressed through the language of mathematics and physical science, moreover, are at our disposal, later we will continue to know other important examples where we can know some essential physical variables through any type of mathematical formulation.

Until another opportunity my dear friends.

Note: The image was created by the author using Power Point and Paint.

Recommended bibliographic references

[1] Hydrostatics. Link.

[2] Hydrostatic Pressure. Link..