Finding Radius of Ex-circle Of a Triangle.

Helo math bugs(🐞) and hivers(🐝)

Well come to another intersting gemetric concept. Here I am going talk about Ex. Here Ex means Exo or outer and thus ex-centre become outer centre of a triangle.

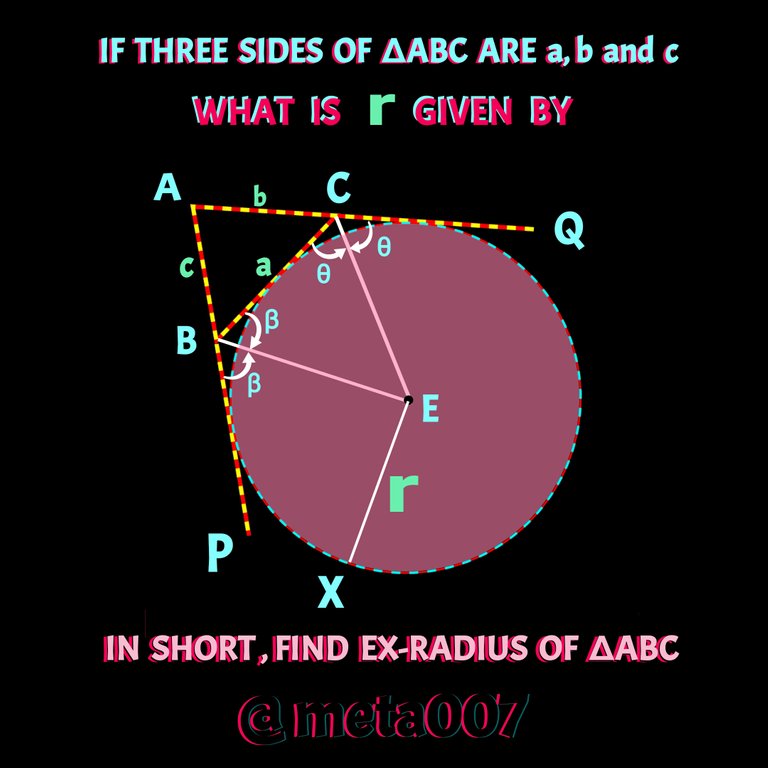
The three sides of ∆ABC are AB, BC and AC. Here in the following picture the O centric circle touches the three sides of ∆ABC. The radius of the circle is called ex-radius. We need two know that the external bisectors of angle B and C will meet at the ex-centre.

There will three ex-centres and three radius.We will just prove one of them and other two can be proved the same say. We are going to prove the ex-radius on not extended side BC and we call it simply r.

We need a simple construction also; let's connect Vertex A and ex-centre E. Check the following figure also.let's also draw the height of ∆ABE and ∆ACE. Each case the height will be r.

Note: For a triangle the height is always perpendicular distance between a vertex and it's opposite side. Hence, a height of a triangle can be outside of the triangle. Here r is the height as a radius is always perpendicular to the point of contact of a tangent.

Let's now concentrate how we can find the area of ∆ABC taking the known variables a,b,c and r. Area of ∆ABE can be given by cr/2 unit² and that of ∆ACE be br/2 and for ∆BEC it will be ar/2. Let's check them below: 👇

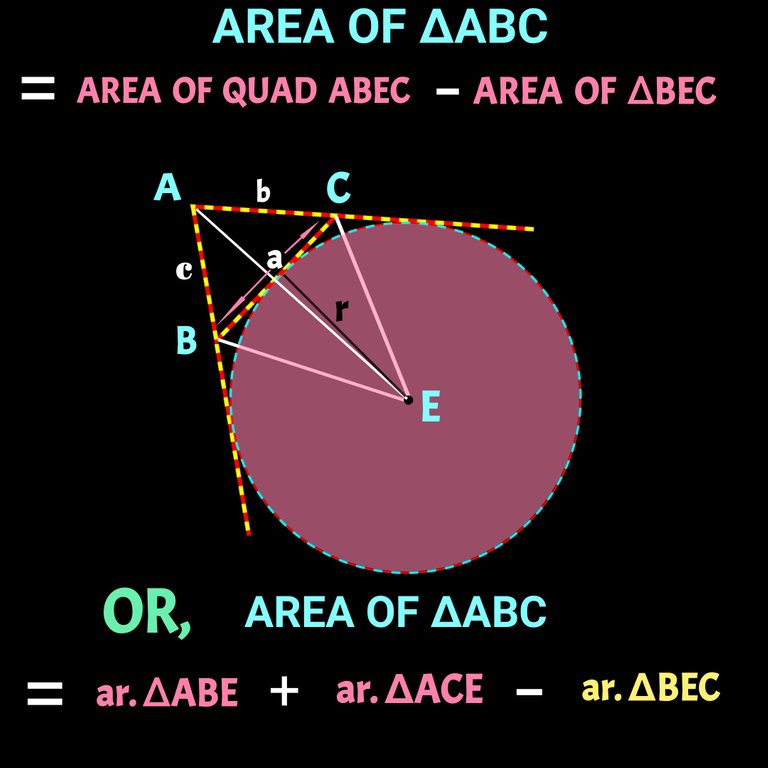
Now we can find the area of ∆ABC.
So, ar.∆ABC = ar. ∆ABE + ar. ∆ACE - ar. ∆BEC. Check it below: 👇

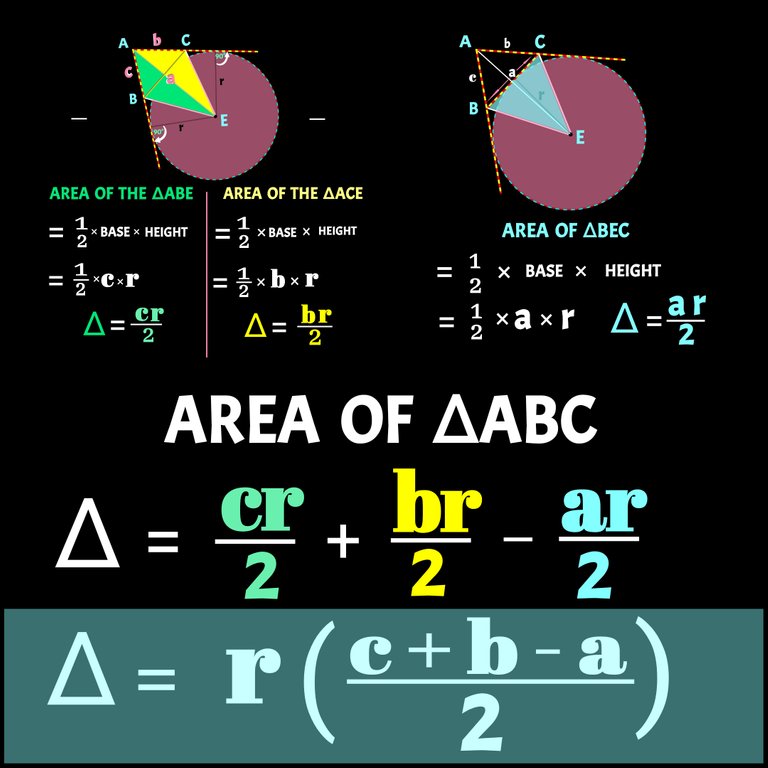
Now time for further calculation. We can write the radius of ex-centre in the form r= ∆/(s-a). Lets check it below: 👇

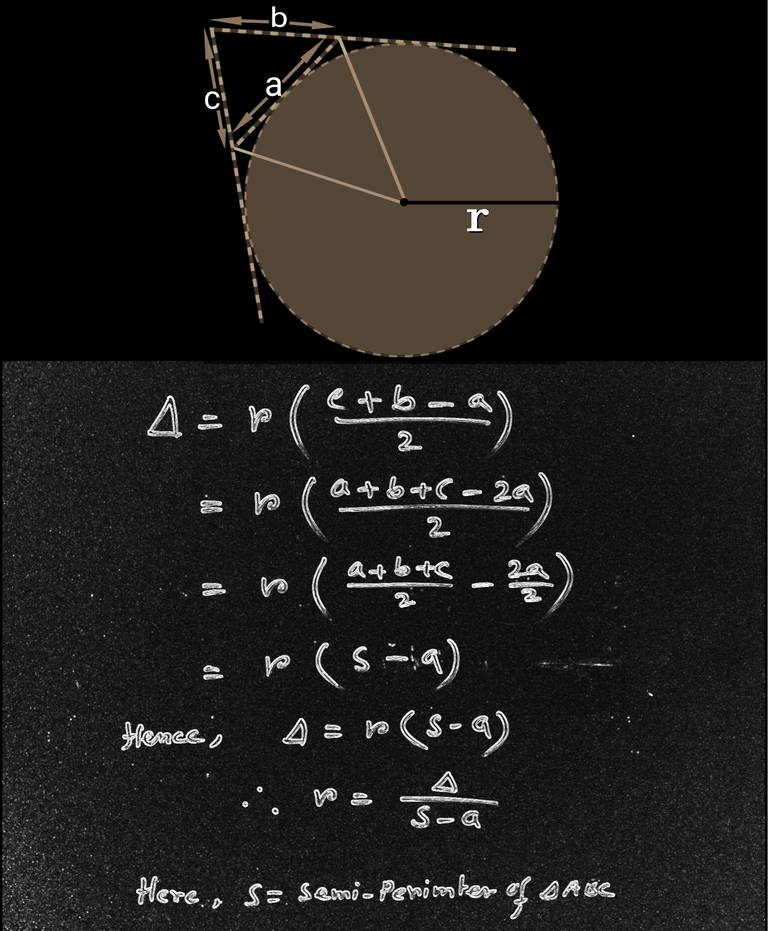
There will three ex-circles and their ex-radius can be given by

r' = ∆/(s-a) unit²
(Radius is perpendicular to not extended side a)

r'' = ∆/(s-b) unit²
(Rdaius is perpendicular to not extended side b)

r''' = ∆/(s-c) unit²
(Radius is perpendicular to not extended side c)

These formullae of radius is very similar to the general one which is given by r = ∆/s , Here r = inradius not the ex one. You can check it here.

🎤🎤All the figures used here is made by me using android application. If you find any silly mistake(s), try ignoring it and just consider the data as the figure may not be accurate in measurement.

Thanks you so much for visiting.

I hope you find my post interesting and effortful.

Have a good day.

All is well

Regards: @meta007