Solving a special system of equations / Resolviendo un sistema de ecuaciones un tanto particular [ EN / ES ]
(Edited)
| English | Español |
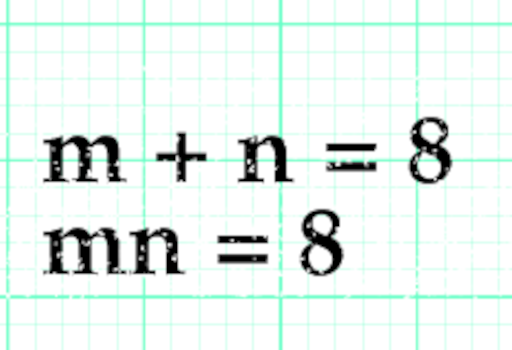
This problem shows a nice property, the equations are symmetric1 functions.
It's well know that the coefficients of a polynomial are symmetric functions of its own roots, so take the quadratic,
x² - (x1 + x2)x + (x1x2) = 0
We can transform our system of equations into a quadratic equation,
x² - 8x + 8 = 0
Solving, by completing the square,
x² - 8x = -8
x² - 8x + 16 = -8 + 16
x² - 8x + 16 = 8
(x - 4)² = 8
x - 4 = ± 2√2
x = 4 ± 2√2
x² - 8x + 16 = -8 + 16
x² - 8x + 16 = 8
(x - 4)² = 8
x - 4 = ± 2√2
x = 4 ± 2√2
whence,
m = 4 + 2√2
n = 4 - 2√2
m = 4 - 2√2
n = 4 + 2√2
the two solutions of the problem at hand.
1Symmetric function:
A function is symmetric if the exchange of its variables does not alters its value⤍| Español | English |
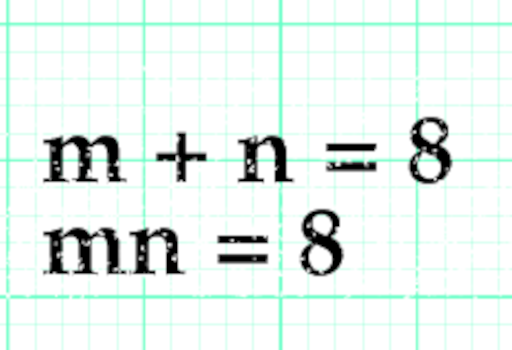
Este problema presenta una particularidad determinante, ya que las funciones que forman el sistema de ecuaciones lo son de un tipo muy especial, ya que son funciones simétricas1.
Es conocido que los coeficientes de un polinomio son funciones simétricas de las raices del mismo, así en el caso del polinomio de segundo grado,
x² - (x1 + x2)x + (x1x2) = 0
Podemos transformar el sistema de ecuaciones dado en una ecuación de segundo grado,
x² - 8x + 8 = 0
La resolvemos, por ejemplo, completando el cuadrado,
x² - 8x = -8
x² - 8x + 16 = -8 + 16
x² - 8x + 16 = 8
(x - 4)² = 8
x - 4 = ± 2√2
x = 4 ± 2√2
x² - 8x + 16 = -8 + 16
x² - 8x + 16 = 8
(x - 4)² = 8
x - 4 = ± 2√2
x = 4 ± 2√2
Por lo tanto,
m = 4 + 2√2
n = 4 - 2√2
m = 4 - 2√2
n = 4 + 2√2
son las dos soluciones del problema planteado.
1Función simétrica:
Una función es simétrica si intercambiando sus variables su valor no se ve alterado ⤍
0
0
0.000
Congratulations @j2e2xae! You have completed the following achievement on the Hive blockchain And have been rewarded with New badge(s)
Your next target is to reach 100 upvotes.
You can view your badges on your board and compare yourself to others in the Ranking
If you no longer want to receive notifications, reply to this comment with the word
STOPCheck out our last posts: