Understanding Projectiles and related concepts

Introduction

In the last course, I looked at a very important type of motion called Simple harmonic Motion. It was well-explained including some fundamental concepts surrounding it. In this article, we will look at another type of motion very important in the world of physics. It is called Projectile motion. First, we will need to understand what a projectile is and then look into the nature of projectile motion.

To begin, here is a definition of the word projectile by Wikipedia:

A projectile is an object that is propelled by the application of an external force and then moves freely under the influence of gravity and air resistance. source

So basically, a projectile involves an object set in motion from rest by the application of some initial force and allowing the object to travel through space until the strength of its force gradually reduces to zero and the object hits the ground or its target.

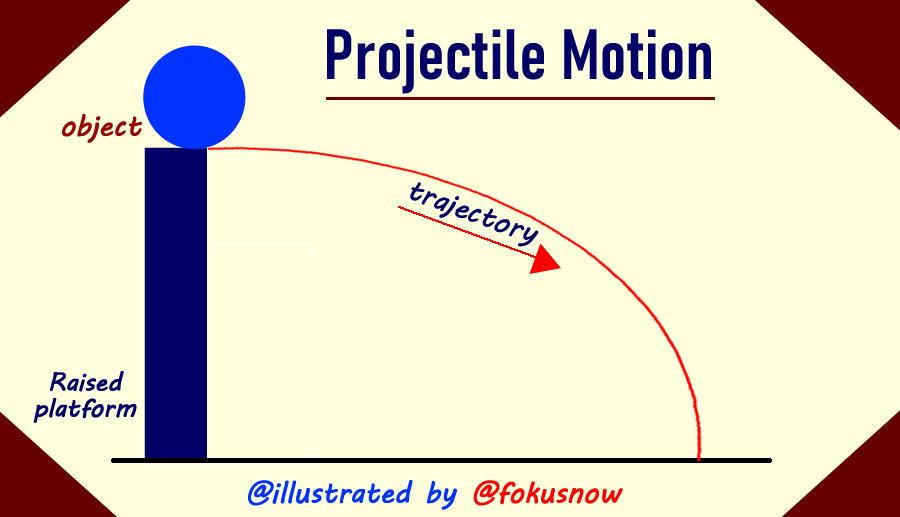
While in motion, projectiles often follow a curved path as the strength of its initial force carries it towards the surface or target. The path of motion of a projectile is often called a trajectory and it is a parabolic path. Once launched, a projectile is often under the influence of the force of gravity which tends to pull it down to the earths surface. The gravitational force often determine the vertical motion of the projectile

The second force that determines how far a projectile could go is the air resistance. How strong the air resistance is affects the horizontal motion of the projectile. So in order to have a well rounded understanding of a projectile and its motion, below are important components to know:

1. Velocity: When an object is projected into space, it starts moving from a state of rest. In order to begin its motion, an initial velocity or force could be applied. It could be manual or mechanical. The velocity of the object in motion generally reduces as it moves through space. Depending on the angle of projection the velocity will eventually reduce to zero, making the object to fall to the ground or hit a target.

2. Motion: A projectile performs both vertical and horizontal motions at the same time. These motions are not related to each other because various forces affect them. For the forward motion, air resistance which is often negligible determines how far they object goes forward. The force of gravity greatly affects its horizontal motion.

3. Trajectory and range: The motion of a projectile often follows a curved path whether it was released from a height or from a horizontal surface. This curved path made by the projectile as it moves is called a trajectory. The range of a projectile is the entire distance it covers from the point where it was released to where it hit the ground.

Examples of projectiles - and calculations

There are many real-world examples of projectiles. They include the following:

• A person jumping off the top of a cliff into water
• Rocket fired from a stationary vehicle on the ground
• Stone thrown from the ground or a height
• Bullet fired from a gun
• Football kicked by a player
• Bomb dropped by a fighter jet

The above are just a few of the many examples of projectiles. You could think of many others if you have understood what a projectile is.

Solving projectile questions

In physics, once could be given a question about the motion of a projectile and they are asked to calculate or look for some missing components of a projectile motion such as height, velocity, etc. To do that is not too difficult. But one thing must be clear.

One needs to understand that a projectile basically makes two types of motion - vertical and horizontal motion. So when a question arises, it could be very easy to isolate the problem and handle of them separately as it relates to either the vertical or horizontal motion of the object. Established equations of motion could be fully applied to solve for unknown variables. So we will demonstrate that right away with just one example.

Example 1: A stone is thrown horizontally from the top of a vertical cliff whose height is 45 meters. If the velocity of the stone is 10ms^-1, calculate the following:

1. How long it took to hit the ground
2. The vertical velocity of the ball when it hit the ground.

Solution
1. How long it took to hit the ground

We will use an equation of motion to handle this, given the known and unknown variables. The equation to use is:

h = ut + 1/2at² where

height h = 45 m
time t = ?
Initial velocity u = 0
Acceleration or velocity a = 10ms^-1

Using h = ut + 1/2at², we have
45 = 0 + 1/2 x 10 x t²
5t² = 45
t² = 45/5
t² = 9 (introducing square root to cancel the square)
t = √9
time = 3 secs

2. The vertical velocity of the ball when it hit the ground.

We will use another equation of motion v = u + gt

Velocity v = ?
Initial velocity u = 0
Acceleration due to gravity g = 10^-1
Time t = 3 secs

v = 0 + 10x3
v = 30^-1

Conclusion

Now we have gotten a good grasp of projectiles and understood the examples used. Projectiles are an important aspect of physics that has practical application in everyday life. In the next lesson, we will see more complex examples.

Reference materials

_{Projectile
What is a Projectile?}