Physics - Classical Mechanics - Viscosity and Turbulence

avatar
(Edited)

[Image1]

Introduction

Hey it's a me again @drifter1!

Today we continue with Physics, and more specifically the branch of "Classical Mechanics", in order to get into Viscosity and Turbulence.

So, without further ado, let's get straight into it!

Real Fluid

In the idealized models we ignored two very important properties of fluid flow, which are viscosity and turbulence. Real fluids differentiate from ideal fluids in exactly these phenomena. Viscosity can be thought of as the internal friction of fluid and turbulence as the mixing and swirling of the various layers caused mainly by obstruction and high speed. Turbulence, more or less, occurs due to the fluid's viscosity, and so these topics are very closely related.

Viscosity

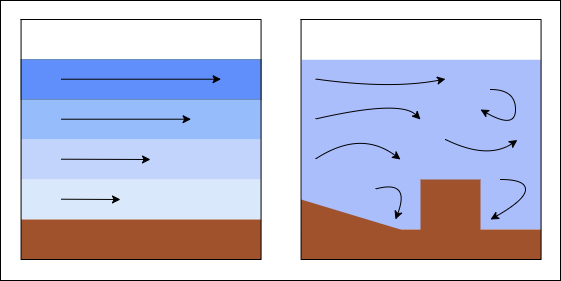
Viscosity is basically the fluid's resistance to flow. The parallel layers of the fluid resist the motion of the fluid layers next to them, as well as the motion of solids that come in contact with them. As such, viscous but non-turbulent flow occurs in layers of different speed. If there was no viscosity the speed would be the same across the whole fluid.

It's due to this resistance of the fluid, also called drag, that the speed at the bottom is much less than the speed at the top. In the context of tubes, due to viscosity, the speed at the walls of the tube is basically zero, and maximum at the center.

If an obstruction comes up or the speed reaches a critical point, the layers start mixing and the flow becomes turbulent.

Measuring Viscosity

In order to end up with a measure of viscosity, we start by placing fluid between two parallel plates. The bottom plate is fixed (v = 0), whilst the top plate moves to the right with a velocity v, dragging the fluid along with it. The layers of fluid that are in contact with the plates don't move relative to these plates. So, the top layer moves at the same speed v as the top plate, and the bottom layer remains at rest.

A visible skew will occur as each layer tries to drag along the next layer and the speed variates from v to 0. The flow is carefully kept laminar, so that the layers don't mix. A continuous shearing motion thus takes place. Because fluids have zero shear strength the rate at which they are sheared is related to the geometrical factors A and L.

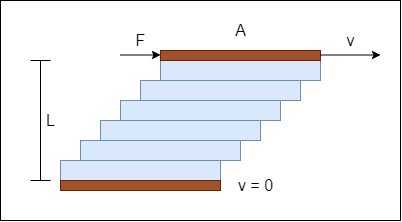
The shear stress between the layers is equal to the force F by the area A. The rate of shearing, also called strain rate or velocity gradient, is the velocity v by the length L. As such, we can now define viscosity as the ratio of these two quantities. Viscosity is represented by the Greek later η (eta) and given by:

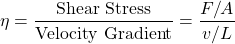
Fluids that satisfy this equation are called Newtonian Fluids, and are a subset of real fluids.

Solving the equation for force gives us:

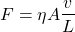
So, the greater the viscosity, the greater the force required.

The S.I. unit of viscosity is the N ⋅ s / m2 or Pa ⋅ s, but its more common to use a quantity related to cgs, the poise, which equals 1 dyn ⋅ s / cm2.

There are actually two types of viscosity. The one measured in poise is also called dynamic viscosity. Dividing it by the density gives us kinematic viscosity which is represented by the Greek letter ν (nu). Kinematic viscosity is measured in stokes (St) which equals 1 cm2 / s. The S.I. unit of 1 m2 / s is rarely used.

Poiseuille’s Law

As we already know, what causes flow is pressure difference. By also taking into account the resistance of fluids to flow, we can derive the following relationship:

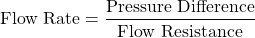
As flow rate we consider the volume flow rate Q = dV / dt. Flow resistance is anything expect pressure that affects flow rate, such as viscosity. It's represented by capital R. As such, if p1 and p2 are the pressures at two points in a tube, the following will be true:

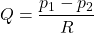
French scientist Poiseuille proved that the resistance to laminar flow in an incompressible fluid with viscosity η through a horizontal tube of radius r and length L is:

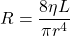
Combining these equations yields Poiseuille’s Law:

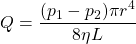
Stokes' Law

Again skipping the proof, let's mention yet another useful equation, which is Stokes' law. It gives us the drag force on a falling sphere:

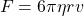
When the sphere is fully submerged and falling with constant velocity, we can derive its viscosity. The buoyant force and drag force are equal to the weight, which gives:

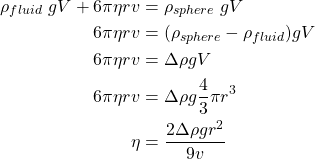
Depending on what's known it's possible to calculate the viscosity (maybe even kinematic) or density of a sphere that is submerged into a liquid.

Turbulence and Reynolds number

Lastly, let's also get into an indicator of turbulence. For a uniform tube, Reynolds number NR is given by:

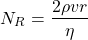
This quantity is dimension-less but experiments revealed that the flow is laminar for values below 2000, and turbulent for values above 3000. In-between values show unstable and chaotic behavior.

RESOURCES:

References

  1. https://courses.lumenlearning.com/suny-osuniversityphysics/chapter/14-7-viscosity-and-turbulence/
  2. https://physics.info/viscosity/

Images

  1. https://pxhere.com/en/photo/1045542

Mathematical equations used in this article, where made using quicklatex.

Visualizations were made using draw.io.

Previous articles of the series

Rectlinear motion

Plane motion

Newton's laws and Applications

Work and Energy

Momentum and Impulse

Angular Motion

Equilibrium and Elasticity

Gravity

Periodic Motion

Fluid Mechanics

Final words | Next up

And this is actually it for today's post!

Next time we will get into Exercises around Fluid Dynamics...

See ya!

Keep on drifting!

Posted with STEMGeeks

physics science education mechanics fluids viscosity turbulence poiseuille stokes stem
0
0
0.000
5 comments
avatar

another great post, thanks for sharing!
!1UP

0
0
0.000
Reply
avatar

The people doing V2K with remote neural monitoring want me to believe this lady @battleaxe is an operator. She is involved deeply with her group and @fyrstikken . Her discord is Battleaxe#1003. I cant prove she is the one directly doing the V2K and RNM. Doing it requires more than one person at the least. It cant be done alone. She cant prove she is not one of the ones doing it. I was drugged in my home covertly, it ended badly. They have tried to kill me and are still trying to kill me. I bet nobody does anything at all. Ask @battleaxe to prove it. I bet she wont. They want me to believe the V2K and RNM in me is being broadcast from her location. And what the fuck is "HOMELAND SECURITY" doing about this shit? I think stumbling over their own dicks maybe? Just like they did and are doing with the Havana Syndrome https://ecency.com/fyrstikken/@fairandbalanced/i-am-the-only-motherfucker-on-the-internet-pointing-to-a-direct-source-for-voice-to-skull-electronic-terrorism

0
0
0.000
Reply
avatar

One question 🙋‍♂️
What if the fluid isn't Newtonian. Is the expression for viscosity still valid or did we need to modify it.

0
0
0.000
Reply
avatar

We have to apply different math for fluids that don't satisfy the viscosity equation. I haven't dug too much into it though...

From it's definition the equation that we use for viscosity isn't a fundamental law, but something like Hooke's law or Ohm's law, which only relates physical quantities with each other. Think of it as an approximation. If we don't need to much accuracy in our measurements and calculations it's "good enough".

0
0
0.000
Reply