Physics - Classical Mechanics - Exercises on Fluid Statics (part 1)
[Image1]
Introduction
Hey it's a me again @drifter1!
Today we continue with Physics, and more specifically the branch of "Classical Mechanics", in order to get into Exercises on Fluid Statics. This is part 1, where we will only cover density and pressure.
So, without further ado, let's get straight into it!
Density Examples
Let's fill out the following table.
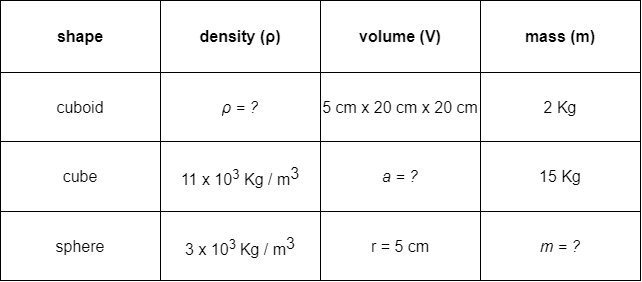
Applying the definition of density ρ = m / V and knowing 3D Geometry it's a piece of cake.
Cuboid
First of all, the volume of the cuboid is 2000 cm3 or 2 x 10-3 m3, because 1 cm3 equals 1 x 10-6 m3.
Therefore, the density is:
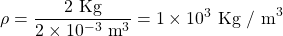
Cube
The volume of the cube is:
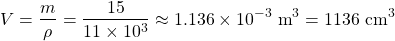
and thus the length of each edge is approximately:
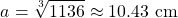
Sphere
The volume of the sphere can be calculated from its radius as follows:
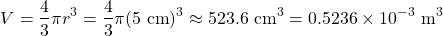
and so the mass is:
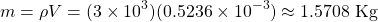
Pressure Examples
Next up, let's calculate the pressure in fluids for various cases.
Below the Ocean
Calculate the Absolute pressure (p) and Gauge pressure (pg) at 4 km below the ocean. The density of seawater is 1.03 x 103 Kg/m3. Suppose an atmospheric pressure (patm) of 1.013 x 105 Pa and acceleration of gravity (g) of 9.8 m/s2.
All quantities are known, and so the absolute pressure is a simple substitution:

For gauge pressure we need to subtract the atmospheric pressure from that result, yielding:
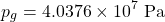
Water Barrel with Oil Layer
Consider a water barrel with a layer of oil on top. The layer of oil is 10 cm thick, whilst the barrel has an overall depth of 60 cm. The density of water is about 1 x 103 Kg/m3. For the oil suppose a density of 0.7 x 103 Kg/m3.
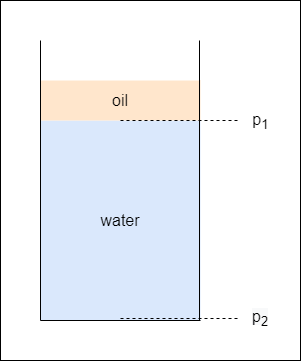
Calculate the gauge pressure at:
- the oil-water interface, and
- the bottom of the barrel
At the oil-water interface only the weight of the oil adds to the pressure. In other words, the gauge pressure is:

At the bottom of the barrel, both the oil and the water need to be considered. The pressure at the top of the water is equal to that at the bottom of the oil, and so 686 Pa. The height of the water "layer" is 60 - 10 = 50 cm. And so the gauge pressure at the bottom is:

Manometer
Consider a manometer in the form of U-tube. One side is open to atmosphere, whilst pressure is applied to the other. The manometer is filled with mercury, which has a density of about 13.6 g/cm3.
For a difference in height between the two columns of 10 cm, calculate the applied pressure.
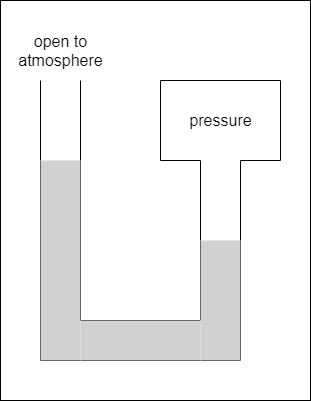
The difference in height indicates the pressure. All the quantities are known, and so the pressure can be calculated directly from ρgh.
Let's calculate only the gauge pressure:
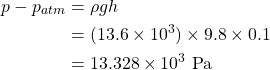
RESOURCES:
Images
Mathematical equations used in this article, where made using quicklatex.
Visualizations were made using draw.io.
Previous articles of the series
Rectlinear motion
- Velocity and acceleration in a rectlinear motion -> velocity, acceleration and averages of those
- Rectlinear motion with constant acceleration and free falling -> const acceleration motion and free fall
- Rectlinear motion with variable acceleration and velocity relativity -> integrations to calculate pos and velocity, relative velocity
- Rectlinear motion exercises -> examples and tasks in rectlinear motion
Plane motion
- Position, velocity and acceleration vectors in a plane motion -> position, velocity and acceleration in plane motion
- Projectile motion as a plane motion -> missile/bullet motion as a plane motion
- Smooth Circular motion -> smooth circular motion theory
- Plane motion exercises -> examples and tasks in plane motions
Newton's laws and Applications
- Force and Newton's first law -> force, 1st law
- Mass and Newton's second law -> mass, 2nd law
- Newton's 3rd law and mass vs weight -> mass vs weight, 3rd law, friction
- Applying Newton's Laws -> free-body diagram, point equilibrium and 2nd law applications
- Contact forces and friction -> contact force, friction
- Dynamics of Circular motion -> circular motion dynamics, applications
- Object equilibrium and 2nd law application examples -> examples of object equilibrium and 2nd law applications
- Contact force and friction examples -> exercises in force and friction
- Circular dynamic and vertical circle motion examples -> exercises in circular dynamics
- Advanced Newton law examples -> advanced (more difficult) exercises
Work and Energy
- Work and Kinetic Energy -> Definition of Work, Work by a constant and variable Force, Work and Kinetic Energy, Power, Exercises
- Conservative and Non-Conservative Forces -> Conservation of Energy, Conservative and Non-Conservative Forces and Fields, Calculations and Exercises
- Potential and Mechanical Energy -> Gravitational and Elastic Potential Energy, Conservation of Mechanical Energy, Problem Solving Strategy & Tips
- Force and Potential Energy -> Force as Energy Derivative (1-dim) and Gradient (3-dim)
- Potential Energy Diagrams -> Energy Diagram Interpretation, Steps and Example
- Internal Energy and Work -> Internal Energy, Internal Work
Momentum and Impulse
- Conservation of Momentum -> Momentum, Conservation of Momentum
- Elastic and Inelastic Collisions -> Collision, Elastic Collision, Inelastic Collision
- Collision Examples -> Various Elastic and Inelastic Collision Examples
- Impulse -> Impulse with Example
- Motion of the Center of Mass -> Center of Mass, Motion analysis with examples
- Explaining the Physics behind Rocket Propulsion -> Required Background, Rocket Propulsion Analysis
Angular Motion
- Angular motion basics -> Angular position, velocity and acceleration
- Rotation with constant angular acceleration -> Constant angular acceleration, Example
- Rotational Kinetic Energy & Moment of Inertia -> Rotational kinetic energy, Moment of Inertia
- Parallel Axis Theorem -> Parallel axis theorem with example
- Torque and Angular Acceleration -> Torque, Relation to Angular Acceleration, Example
- Rotation about a moving axis (Rolling motion) -> Fixed and moving axis rotation
- Work and Power in Angular Motion -> Work, Work-Energy Theorem, Power
- Angular Momentum -> Angular Momentum and its conservation
- Explaining the Physics behind Mechanical Gyroscopes -> What they are, History, How they work (Precession, Mathematical Analysis) Difference to Accelerometers
- Exercises around Angular motion -> Angular motion examples
Equilibrium and Elasticity
- Rigid Body Equilibrium -> Equilibrium Conditions of Rigid Bodies, Center of Gravity, Solving Equilibrium Problems
- Force Couple System -> Force Couple System, Example
- Tensile Stress and Strain -> Tensile Stress, Tensile Strain, Young's Modulus, Poisson's Ratio
- Volumetric Stress and Strain -> Volumetric Stress, Volumetric Strain, Bulk's Modulus of Elasticity, Compressibility
- Cross-Sectional Stress and Strain -> Shear Stress, Shear Strain, Shear Modulus
- Elasticity and Plasticity of Common Materials -> Elasticity, Plasticity, Stress-Strain Diagram, Fracture, Common Materials
- Rigid Body Equilibrium Exercises -> Center of Gravity Calculation, Equilibrium Problems
- Exercises on Elasticity and Plasticity -> Young Modulus, Bulk Modulus and Shear Modulus Examples
Gravity
- Newton's Law of Gravitation -> Newton's Law of Gravity, Gravitational Constant G
- Weight: The Force of Gravity -> Weight, Gravitational Acceleration, Gravity on Earth and Planets of the Solar System
- Gravitational Fields -> Gravitational Field Mathematics and Visualization
- Gravitational Potential Energy -> Gravitational Potential Energy, Potential and Escape Velocity
- Exercises around Newtonian Gravity (part 1) -> Examples on the Universal Law of Gravitation
- Exercises around Newtonian Gravity (part2) -> Examples on Gravitational Fields and Potential Energy
- Explaining the Physics behind Satellite Motion -> The Circular Motion of Satellites
- Kepler's Laws of Planetary Motion -> Kepler's Story, Elliptical Orbits, Kepler's Laws
- Spherical Mass Distributions -> Spherical Mass Distribution, Gravity Outside and Within a Spherical Shell, Simple Examples
- Earth's Rotation and its Effect on Gravity -> Gravity on Earth, Apparent Weight
- Black Holes and Schwarzschild Radius -> Black Holes (Creation, Types, How To "See" Them), Schwarzschild Radius
Periodic Motion
- Periodic Motion Fundamentals -> Fundamentals (Period, Frequency, Angular Frequency, Return Force, Acceleration, Velocity, Amplitude), Simple Harmonic Motion, Example
- Energy in Simple Harmonic Motion -> Forms of Energy in SHM (Potential, Kinetic, Total and Maximum Energy, Maximum Velocity), Simple Example
- Simple Harmonic Motion Equations -> SHM Equations (Displacement, Velocity, Acceleration, Phase Angle, Amplitude)
- Simple Harmonic Motion and Reference Circle -> SHM and Smooth Circular Motion, Reference Circle
- Simple Harmonic Motion Exercises -> 2 Complete Examples on Simple Harmonic Motion
- Simple Pendulum -> Simple Pendulum (Return Force, Small Angle Approximations, More Accurate Period, Gravity Approximation)
- Physical Pendulum -> Physical Pendulum (Return Torque, Small Angle Approximations, Estimating Moment of Inertia)
- Exercises around Pendulums -> Complete Examples on the 2 types of Pendulums (Simple, Physical)
- Damped Oscillation -> Damping Force, Total Force and Differential Equation, Motion Equations, Special Cases
- Forced Oscillation and Resonance -> Forced Oscillation (Differential Equation, Amplitude, Resonance)
- Exercises around Damped and Forced Oscillation -> Complete Examples on Damped Oscillation and Forced Oscillation
- Chaos and Chaotic Oscillation -> Chaos, Unpredictability and Randomness, Chaotic Oscillation
Fluid Mechanics
- Density and Pressure -> Fluids and Fluid Mechanics, Density, Specific Gravity, Pressure
- Measuring Pressure in Fluids -> Pressure in Fluids (Variation with Depth), Absolute and Gauge Pressure, Measuring Pressure
- Pascal's Principle and Hydraulics -> Static Equilibrium, Pascal's Principle, Hydraulic Systems
- Archimedes' Principle and Buoyancy -> Buoyant Force, Archimedes' Principle, Relation with Density
- Surface Tension -> Surface Tension (Cohesive and Adhesive Forces, Unit, Definition), Capillarity
Final words | Next up
And this is actually it for today's post!
Next time we will get into examples around the remaining Fluid Static topics...
See ya!

Keep on drifting!
Posted with STEMGeeks