Mathematics - Discrete Mathematics - Boolean Algebra

over 2 years ago

Introduction

Hey it's a me again @drifter1!

Today we continue with Mathematics, and more specifically the branch of "Discrete Mathematics", in order to get into Boolean Algebra.

This will be a small introduction to the topic. For more information I highly suggest checking out Logic Design in general.

So, without further ado, let's get straight into it!

Boolean Algebra

Boolean algebra is a branch of mathematics, which studies the algebra of 0 and 1, or false and true. It finds many applications in computer science, with logical circuits being the most direct application, but it's also useful in programming / coding in general.

Boolean Operations

The three main boolean operations are:

AND (conjunction)
OR (disjunction)
NOT (negation)

These are the same exact operations that were covered in the article about Propositional Logic.

Notation

In Boolean algebra the AND and OR operations are commonly symbolized using multiplication and addition respectively, meaning that AND uses "*" or even nothing, and OR uses "+". Using the symbols ∧ and ∨ is of course also valid. Lastly, negation is usually represented by an apostrophe (') or an overline (-) instead of an tilde (~) in front of the respective boolean variable.

Boolean Properties

Boolean operations satisfy the properties shown in the table below.

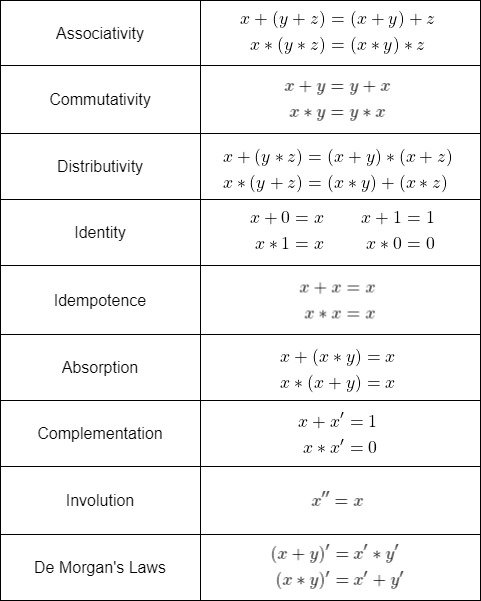
Boolean algebra is basically a complemented, distributive lattice, with boolean operations AND (∧ or *), OR (∨ or +) and a unique complement or unary operation defined for each element. The elements are only two: 0 and 1.

Boolean Expressions

Boolean expressions are expressions defined over boolean Algebra. Each element in such an expression is an boolean expression itself (i.e. 0 and 1 are boolean expressions on their own).

For example:

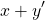
is a valid boolean expressions with boolean variables x and y.

Such expressions are evaluated by assigning either 0 or 1 to each boolean variable, yielding a final result of 0 or 1.

Equivalent Expressions

Of course, there can be multiple boolean expressions giving the same result for the same assignment of values. These so called equivalent expressions have the same truth table.

Canonical Forms

It's common to write boolean expressions in either disjunctive or conjunctive normal form, and so basically as a sum of products (SOP) or a product of sums (POS). In a SOP, the product terms are known as min-terms (resulting in 1), whilst in a POS the sum terms are called max-terms (resulting in 0).

RESOURCES:

References

Images

https://commons.wikimedia.org/wiki/File:Truth_table_for_AND,_OR,_and_NOT.png

Mathematical equations used in this article, have been generated using quicklatex.

Block diagrams and other visualizations were made using draw.io.

Previous articles of the series

Introduction → Discrete Mathematics, Why Discrete Math, Series Outline
Sets → Set Theory, Sets (Representation, Common Notations, Cardinality, Types)
Set Operations → Venn Diagrams, Set Operations, Properties and Laws
Sets and Relations → Cartesian Product of Sets, Relation and Function Terminology (Domain, Co-Domain and Range, Types and Properties)
Relation Closures → Relation Closures (Reflexive, Symmetric, Transitive), Full-On Example
Equivalence Relations → Equivalence Relations (Properties, Equivalent Elements, Equivalence Classes, Partitions)
Partial Order Relations and Sets → Partial Order Relations, POSET (Elements, Max-Min, Upper-Lower Bounds), Hasse Diagrams, Total Order Relations, Lattices
Combinatorial Principles → Combinatorics, Basic Counting Principles (Additive, Multiplicative), Inclusion-Exclusion Principle (PIE)
Combinations and Permutations → Factorial, Binomial Coefficient, Combination and Permutation (with / out repetition)
Combinatorics Topics → Pigeonhole Principle, Pascal's Triangle and Binomial Theorem, Counting Derangements
Propositions and Connectives → Propositional Logic, Propositions, Connectives (∧, ∨, →, ↔ and ¬)
Implication and Equivalence Statements → Truth Tables, Implication, Equivalence, Propositional Algebra
Proof Strategies (part 1) → Proofs, Direct Proof, Proof by Contrapositive, Proof by Contradiction
Proof Strategies (part 2) → Proof by Cases, Proof by Counter-Example, Mathematical Induction
Sequences and Recurrence Relations → Sequences (Terms, Definition, Arithmetic, Geometric), Recurrence Relations
Probability → Probability Theory, Probability, Theorems, Example
Conditional Probability → Conditional Probability, Law of Total Probability, Bayes' Theorem, Full-On Example
Graphs → Graph Theory, Graphs (Vertices, Types, Handshake Lemma)
Graphs 2 → Graph Representation (Adjacency Matrix and Lists), Graph Types and Properties (Isomorphic, Subgraphs, Bipartite, Regular, Planar)
Paths and Circuits → Paths, Circuits, Euler, Hamilton
Trees → Trees (Rooted, General and Binary), Tree Traversal, Spanning Trees
Common Graph Problems → Shortest Path Problem, Graph Connectivity, Travelling Salesman Problem, Minimum Spanning Tree, Maximum Network Flow, Graph Coloring
Binary Operations → Binary Operations (n-ary, Table Representation), Properties
Groups → Groups (Properties, Theorems, Finite and Infinite, Abelian, Cyclic, Product, Homo-, Iso- and Auto-morphism)
Group-like Structures (part 1) → Subgroups, Semigroups, Monoids
Group-like Structures (part 2) → Magma, Quasigroup, Groupoid
Rings → Rings (Axioms, Commutative and Non-Commutative, Semirings, Subrings, Rng)
Fields → Fields (Axioms, Subfields, Finite Fields, Field Extension)