Solving Math Inequalities With Quadratic Equations

Hi there. In this math post, I cover the topic of solving math inequalities with quadratic equations. This topic is algebraic in nature. It is assumed that the reader is comfortable with quadratic equations, the algebra topic of factoring, inequality signs (<, >, ≤, ≥) and working with inequalities.

Math text done with LaTeX rendering through Quicklatex.com.

Pixabay Image Source

 

Topics

---

• Solving Quadratic Inequalities With Factoring Techniques
• Quadratic Inequalities & The Quadratic Formula

 

Solving Quadratic Inequalities With Factoring Techniques

---

Example One

Solve for x in .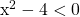

The objective is to find x-values such that the quadratic function is less than 0. First, we factor the quadratic to obtain the x-intercepts for the quadratic.

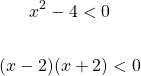
The x-intercepts for the quadratic function are -2 and 2. These x-intercept values make the quadratic function zero. Use the values of -2 and 2 to help find valid values for x that make the inequality true with the use of a table.

x	y
-2	0
-1	-3
0	-4
1	-3
2	0

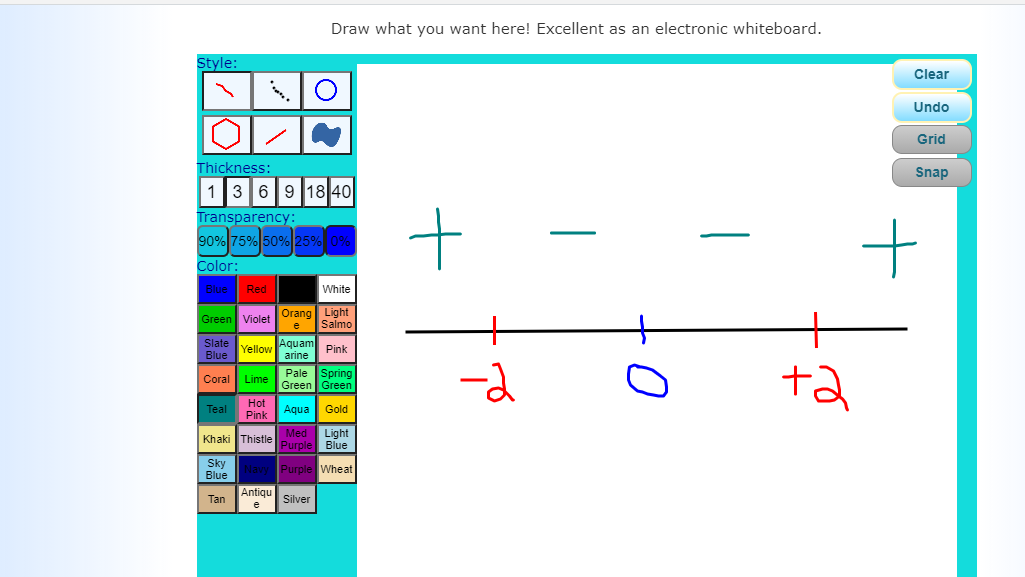
I use a number line type of diagram to determine intervals where the quadratic is positive or negative. I use the test values of -3, 0 and + 3 for determining the sign of the quadratic function.

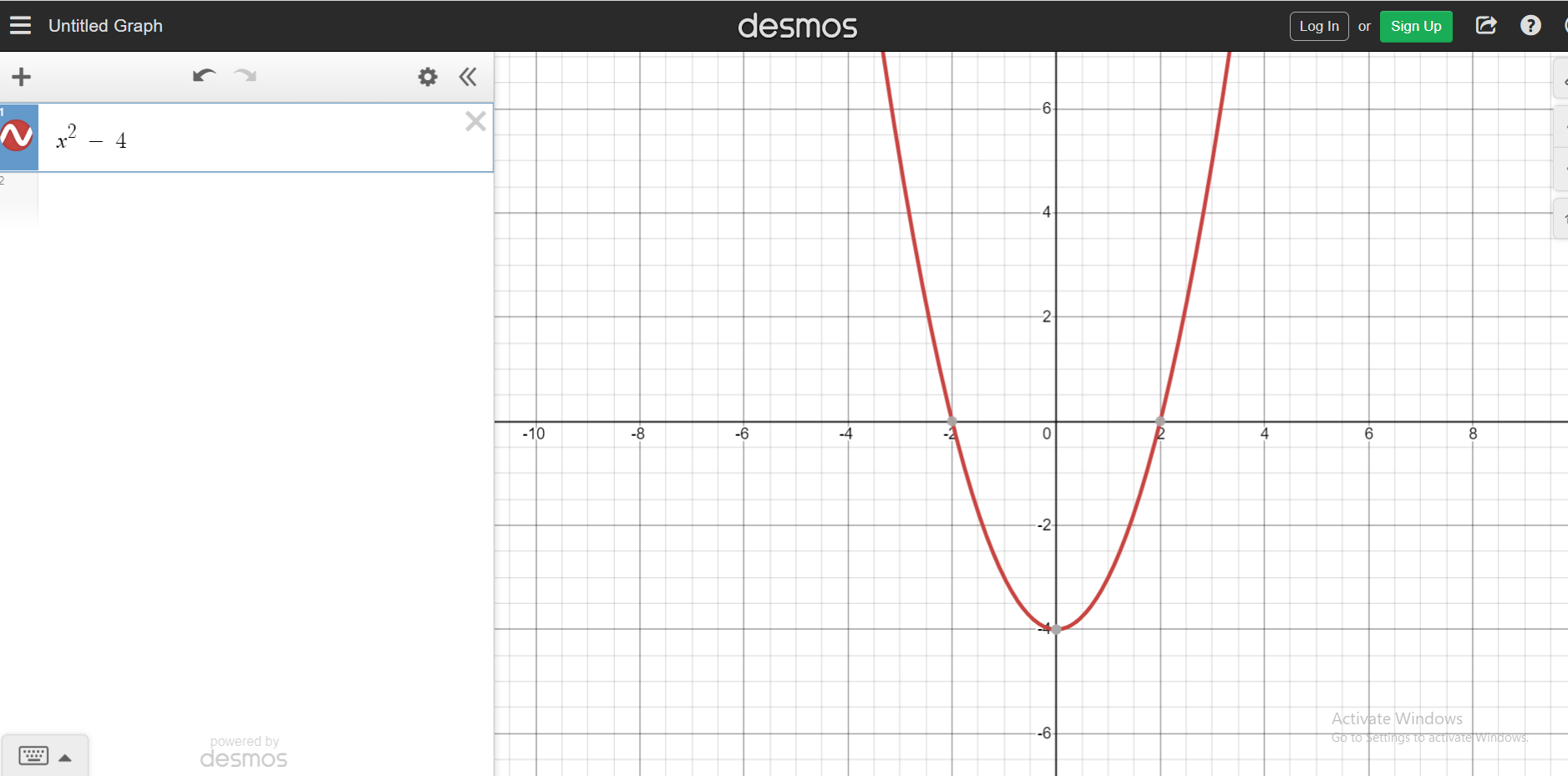
If a graphing tool is allowed you can use Desmos to visualize the solution set.

The quadratic function for this question is negative when x is greater than -2 or when x is less than 2. In math notation this is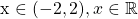

 

Example Two

Solve for x in .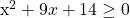

The two factors of 14 that add up together to 9 are 7 and 2. Factoring the left side of the inequality yields:

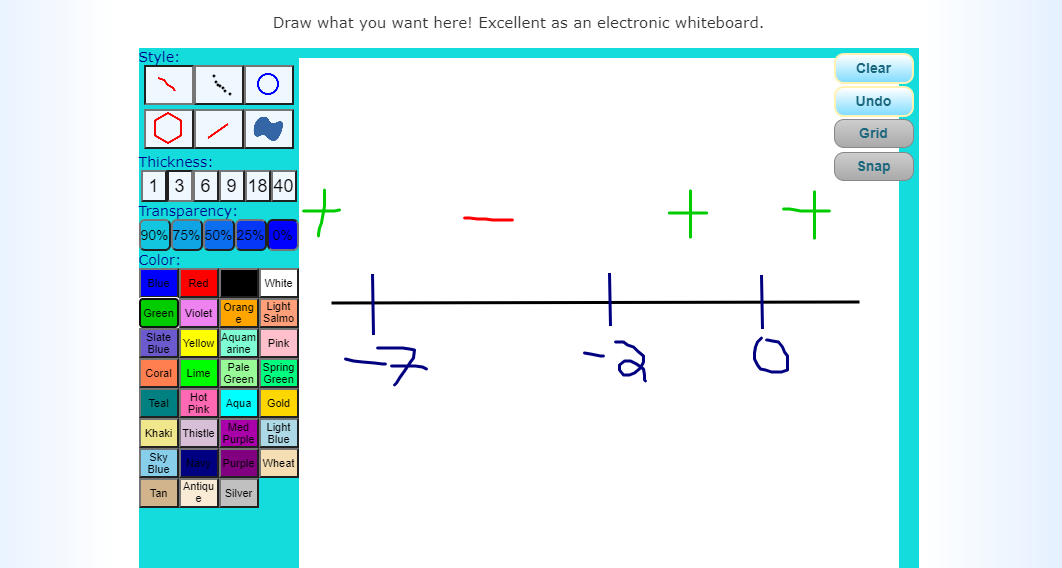
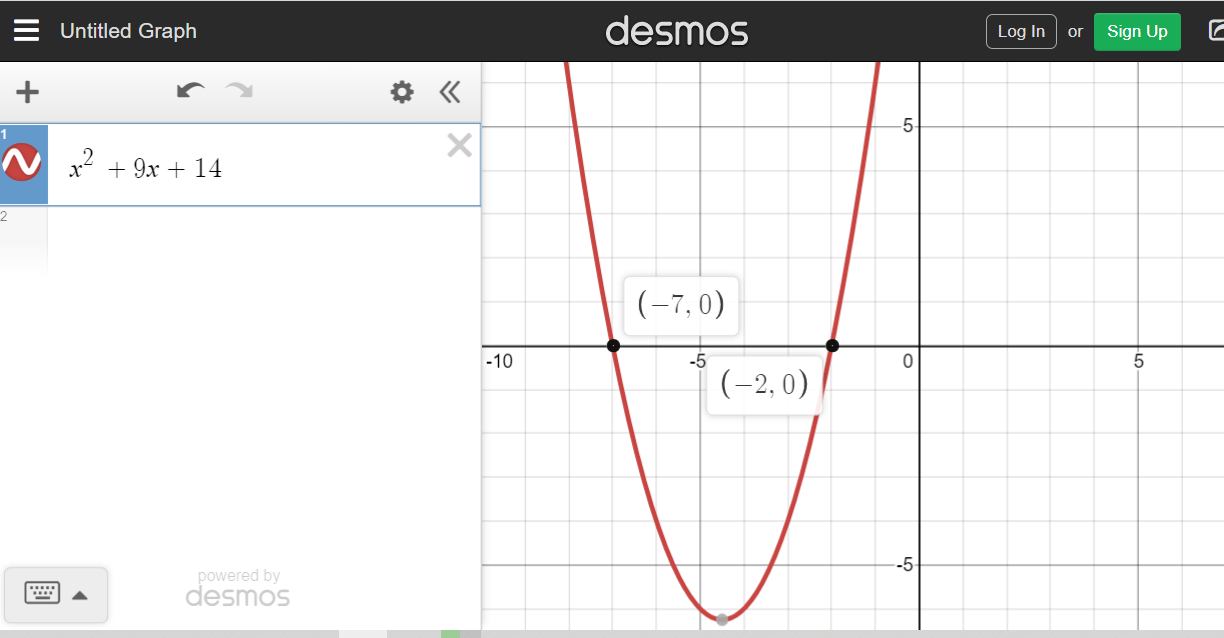
From the left side, the x-intercepts are -7 and -2. Create a table with the values of -7 and -2 as key values.

The solution set is when x is less than or equal to -7 or when x is greater than or equal to -2.

 

Example Three

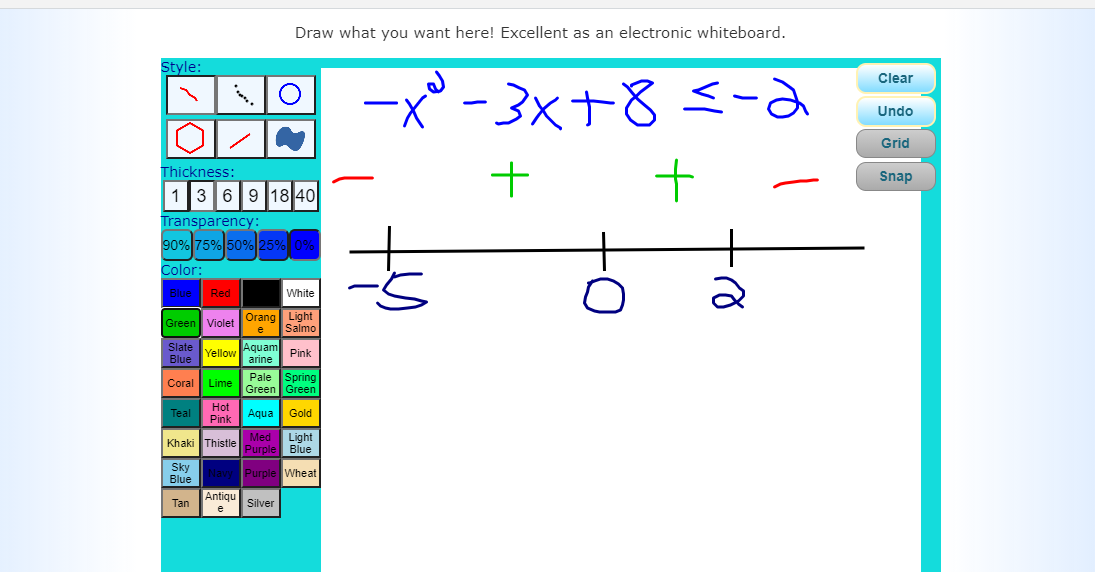
Solve for x in .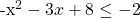

Start with adding both sides by 2. Afterwards factor a negative one as a common factor.

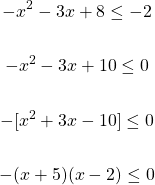
The x-intercepts for the quadratic on the left side are -5 and +2. A table can be created to help identify the intervals where the quadratic is negative or positive. Be careful of the negative sign. The negative sign would change the signs.

The solution set for x in 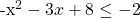is when x is less than or equal to -5 or when x is greater than or equal to 2. In math notation this is

 

Quadratic Inequalities & The Quadratic Formula

---

Not every quadratic can be factored. With non-factorable quadratics, the quadratic formula is needed. As a reference here is the quadratic formula given .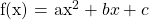

 

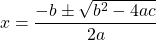
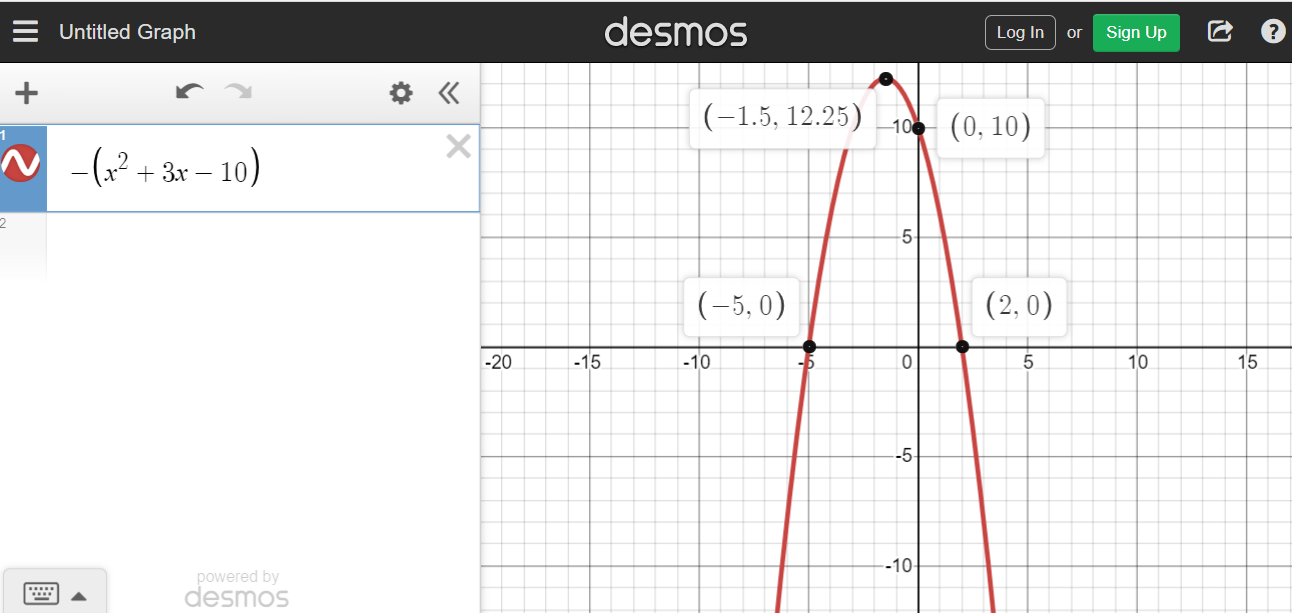
Example

Solve for x in .

fix this

Using the quadratic formula for gives:

 

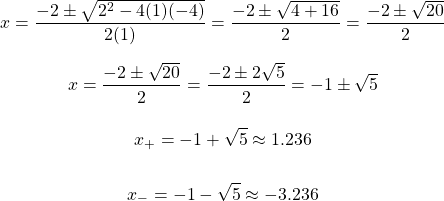
I have decided not to show a table here but have included a Desmos screenshot.

The quadratic is less than 0 when 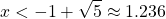or .

Pixabay Image Source

Thank you for reading.

 

Posted with STEMGeeks