Find the equation of the line that is tangent to f(x) at a given point
Hello friends and followers of STEM content.
In this opportunity I am going to explain how we can calculate the equation of the line that is tangent to a real function at a given point.
As data for the exercise we need a function f(x) and the coordinates of a point in the plane, so from these data we will get the equation of the line that is tangent to the function at a given point, then I present the statement of the exercise:
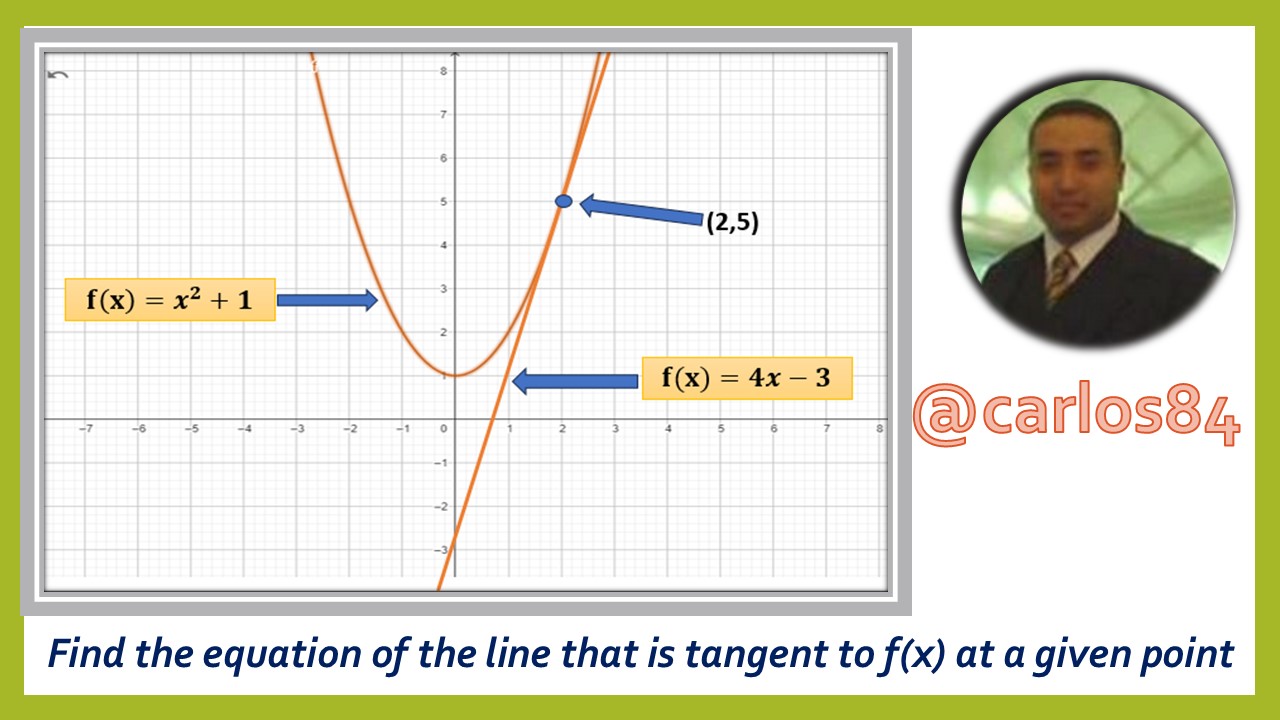
Find the equation of the tangent line to the quadratic function at the point (2,5).
Quadratic function:
- Confirm by means of a graphical representation in Geogebra if the equation of the line is really tangent to the quadratic function at the point (2,5).
Solution approach:
What data do we know about the straight line?
We have a point whose coordinates in the plane are (2,5).
If we knew the coordinates in the plane of another point belonging to the line, we could then find its slope, and with the coordinates of a point and the slope we can apply the equation point - slope and find the equation of the line, but since we do not have the coordinates of another point other than (2,5), we have to find another solution to get the slope of the tangent line to the quadratic function.
To find the slope of the line that is tangent to the quadratic function at the point (2,5) we must understand the geometric death of the Derivative.
Geometrically the derivative is defined as the slope of the tangent line to any continuous function at a given point.
Given the above definition, we can deduce that, if we find the derivative of the quadratic function at the point (2.5) we would be finding the slope of the tangent line at the point (2.5).
How can we find the derivative of the quadratic function?
The first thing to do is to analyze the quadratic function to know how many terms the function is made up of, as you can see it has two terms:
First term:
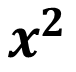
Second term:
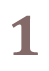
As it has several terms, we derive each of the terms, so the following approach would remain:
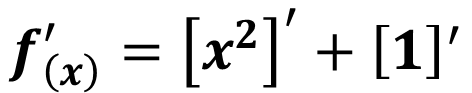
When we derive 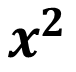 we must lower the exponent, which in this case is 2, and we must subtract 1 from the exponent 2, so it would be 2x, as shown:
we must lower the exponent, which in this case is 2, and we must subtract 1 from the exponent 2, so it would be 2x, as shown:

The derivative of a constant (any number) is equal to zero, therefore from the second term its derivative would be:
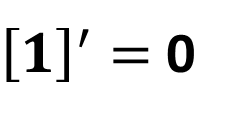
If we make the substitution, the derivative of the quadratic function would be:
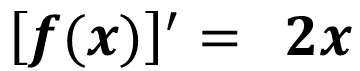
It only remains to substitute the value of the x-coordinate of the point (2,5) in the derivative of the quadratic function and we find the slope (m) of the line that is tangent to the quadratic function at the point (2,5):
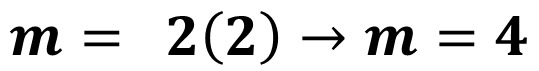
Therefore of the line that is tangent to the quadratic function at the point (2,5) we know the slope m=4 and the point (2,5), so to get the equation of the line we can use the equation point - slope:
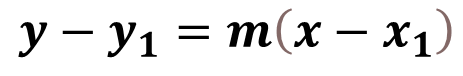
Where x1 is, we substitute the value of 2, and where y1 is, we substitute the value of 5, and where m is, we substitute the value of 4, so we would be left with
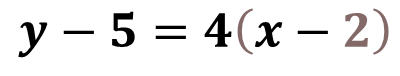
Applying the distributive property, clearing and simplifying everything, we would be left with:

The equation of the line that is tangent to the quadratic function at the point (2,1) is:
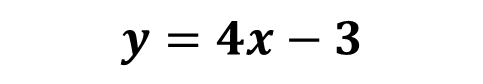
Can we graph the line and the parabola and verify that the line is the tangent of the parabola at the point (2,5)?
The next procedure is to be able to graph the line and the parabola and be able to see if the line is tangent to the quadratic function at the point (2,5):
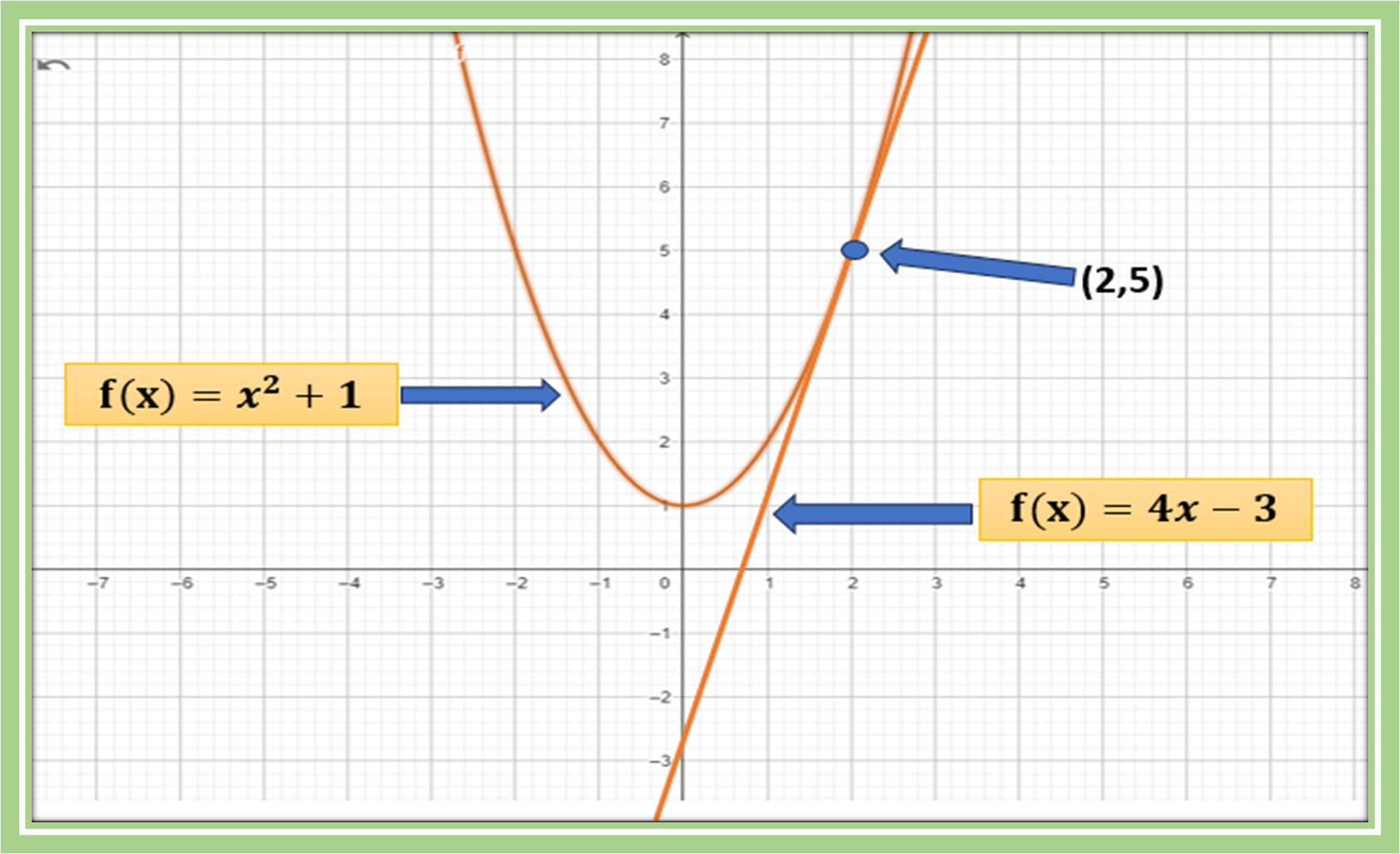
The graph shown can demonstrate how the equation of the line is tangent to the quadratic function at the point (2,5).
Recommended bibliography
Calculus with Analytic Geometry. Volume I. 7th edition. Author: Larson and Hostetler.
Note: All equations in this post were prepared using Microsoft PowerPoint equation insertion tools. The graph of the rational function was made using geogebra software.
Thanks for your contribution to the STEMsocial community. Feel free to join us on discord to get to know the rest of us!
Please consider delegating to the @stemsocial account (85% of the curation rewards are returned).
You may also include @stemsocial as a beneficiary of the rewards of this post to get a stronger support.